假设检验——《概率论与数理统计》第八章学习笔记

前言

感谢台风暹芭，让我回不去宿舍，被迫在实验室过夜，思来想去，睡不着，恰逢期末考临近，决定写一篇的第八章的学习笔记。

和之前的系列一样，教材不变。内容上，选取第八章的前三节，即假设检验，正态均值，正态方差三个部分的知识点，为什么没有其他内容，因为这次考试大概不会考。

形式上，相比前面的章节写了很多课本的定义，这次我会有更多的个人理解，尽可能直击考点。

MindMap

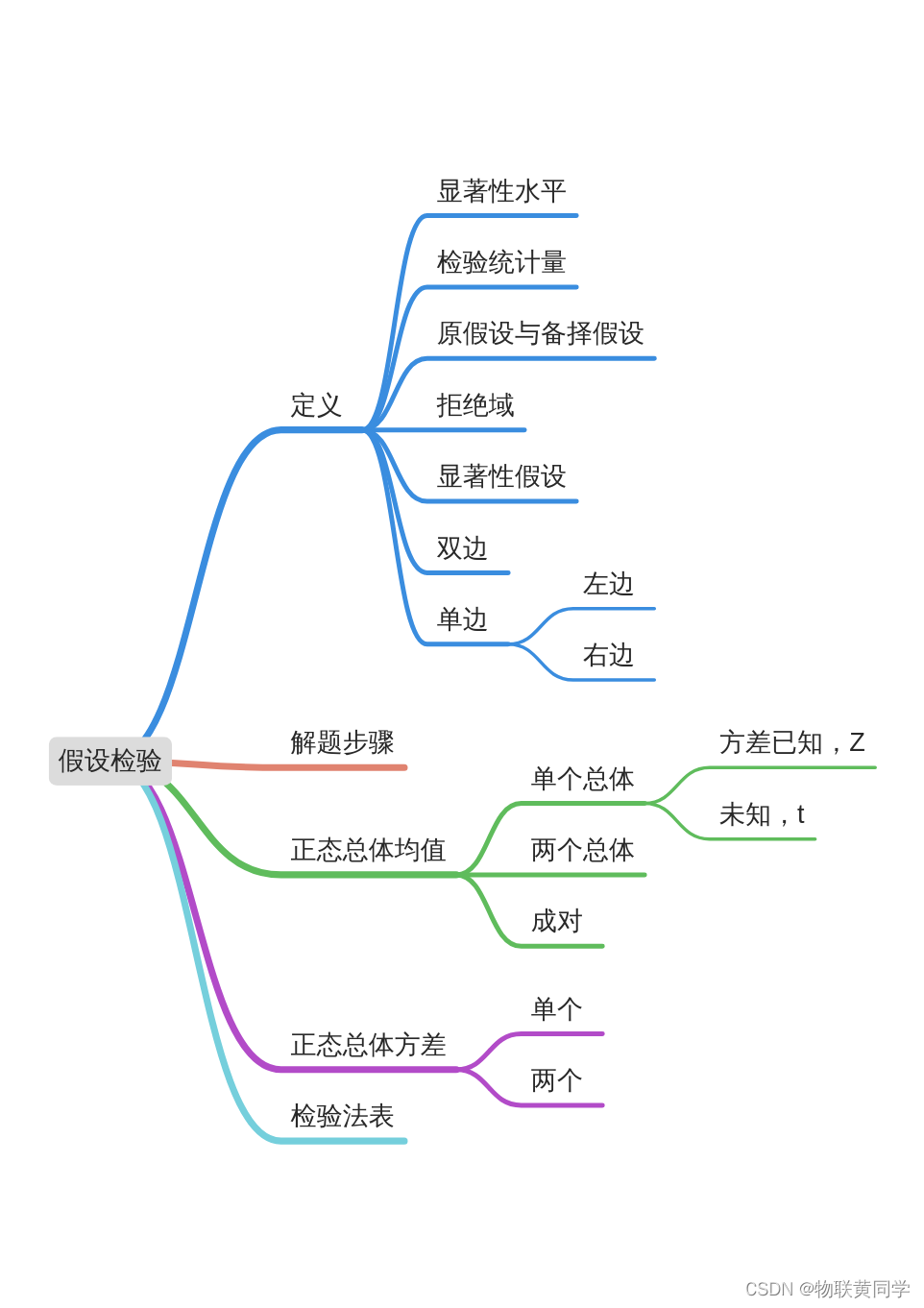
假设检验

这一节其实就是告诉你假设检验的一些定义，以及对假设检验这一问题的解决过程与步骤。

一些定义

显著性水平

我们用来对检验做衡量的标准，一般在式子中以 α出现。

检验统计量

$$Z=\frac{\overline{X}-{\mu }_0}{\sigma /\sqrt{n}}$$

原假设 与 备择假设

我们将检验问题叙述成：在显著性水平α下，检验假设：
$$H_0:\mu ={\mu }_0,H_1:\mu \ne {\mu }_0$$
H0 为 原假设， H1 为 备择假设。

拒绝域

就是在某个区域上取值作为 检验统计量的值时，拒绝 原假设，或者说接受备择假设，这个区域就是拒绝域，而拒绝域的边界点其实就叫 临界点。

显著性检验

因为检验的依据是样本，所以检验势必会有犯错的可能，这里主要有两种错误：

1. H0为真，但是拒绝。
2. H1为真，但是接受原假设。

我们显然希望犯这两种错误的概率都小，但是在数理统计中，如果样本容量限定了，则减小犯一类错误的概率减小的同时，另一类的概率往往增大。所以在数理统计中，采取的是对第一类控制，不考虑 第二类。这种检验就是 假设性检验。

双边检验与单边检验

这里其实就是我们在做假设的时候，对于H1，μ可能大于μ0，有可能小于μ0，如果是两种都可能，那就是 双边假设， 而如果只是其中一种可能，那就是 单边假设，根据方向又可以分为 左边检验 和 右边检验。对于方向，我的个人理解是看 拒绝域或者备择假设的方向。

个人理解的解题步骤

通过阅读与理解课本的例题，发现了假设检验问题的求解过程：

1. 先根据题目确定检验假设。
2. 根据参数确定检验统计量。
3. 然后根据假设和检验统计量判断是那种假设，然后确定拒绝域。
4. 取样，其实就是根据样本观察值判断是否接受原假设。

本篇中所有的总体都是正态总体，针对它的两个参数，均值μ和方差σ^2，有以下两种假设检验。

正态总体均值的假设检验

单个总体

这里根据方差是否已知，又可以分为 Z检验 和 t检验。

方差已知，Z检验

其实很简单，我们根据假设然后接下来需要检验样本均值是否符合假设，在显著性水平α以及其他参数下，检验统计量为：
$$\begin{gathered} Z=\frac{\overline{X}-{\mu }_0}{\sigma /\sqrt{n}} \\ Z\sim N(\mu ,{\sigma }^2) \end{gathered}$$
接下来只需根据是单边假设还是双边假设来求解就可以了。

双边就取α/2，检验统计量的绝对值高于 显著性水平下对应的 正态函数值就拒绝原假设。

方差未知，t检验

这里其实就是 用了样本方差s 来近似替换 总体方差σ，当然这里需要用到t分布。
$$\frac{\overline{X}-{\mu }_0}{S/\sqrt{n}}\sim t(n-1)$$

两个总体——t检验

我们对两个独立的正态总体
$$N({\mu }_1,{\sigma }^2),N({\mu }_2,{\sigma }^2)$$
方差相同，均值不同，所以可以剔除检验假设：
$$H_0:{\mu }_1-{\mu }_2=\delta ,H_1:{\mu }_1-{\mu }_2\ne \delta$$
所以给出检验统计量：
$$\begin{gathered} t=\frac{(\overline{X}-\overline{Y})-\delta }{S_w\sqrt{\frac{1}{n_1}+\frac{1}{n_2}}} \\ {S}_w^2=\frac{(n_1-1)S_1^2+(n_2-1)S_2^2}{n_1{n}_2-2} \end{gathered}$$

成对数据的检验——t检验

其实这里就是将两组数据对比求差异，然后做检验，我们一般是直接将数据相减后作为一个新的正态总体样本，接下来其实就是单个总体下的情况了。

正态总体方差的假设检验

单个总体

在均值中，我们用到的是Z和t检验，说白了就是用到正态分布和 t分布, 但是在求方差的假设检验的时候，其实用到的是
$$\frac{(n-1)S^2}{{\sigma }_0^2}\sim {\chi }^2(n-1)$$

两个总体

用到的是F分布
$$\frac{S_1^2/S_2^2}{{\sigma }_1^2/{\sigma }_2^2}\sim F(n_1-1,n_2-1)$$

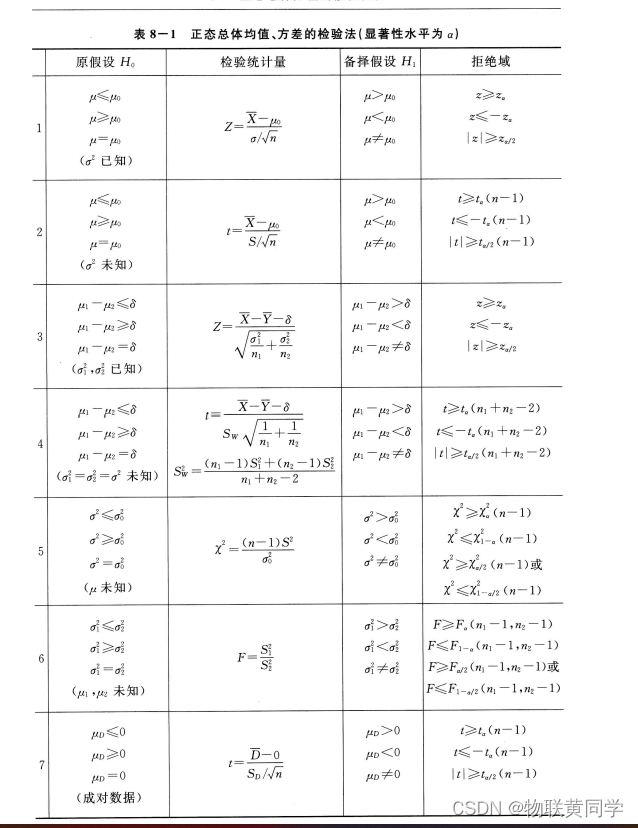
正态总体的检验法表

后话

天亮了，回去睡觉了，这一章结合教材阅读会更好一点。