当前位置:网站首页>A brief introduction to heading/pitch/roll and omega/phi/kappa
A brief introduction to heading/pitch/roll and omega/phi/kappa
2022-07-05 06:37:00 【Flying from place to place】
Heading/Pitch/Roll vs Omega/Phi/Kappa
I am engaged in aerial triangulation , These two sets of attitude information are often used , Some information checked on domestic and foreign websites , I've sorted out .
Heading/Pitch/Roll
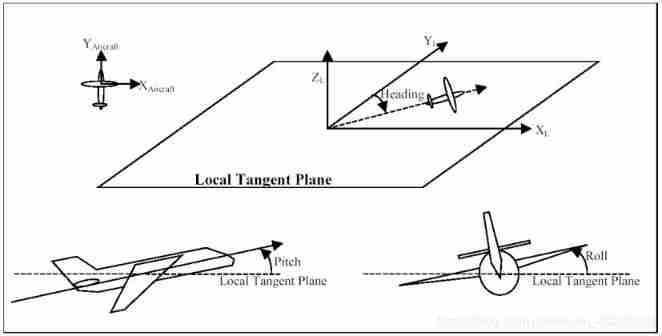
The first said Heading, Pitch, and Roll. These are the images / Sensors x、y、z The axis is transformed into three rotations of the local horizontal plane . Generally speaking , These rotations are applied sequentially .
Heading - around Z The rotation of the shaft .
Pitch - around X Shaft rotation
Roll - Around the Y Shaft rotation
Heading、Pitch、Roll It's the plane ( That's the image ) Of the local plane X、Y、Z The angle of the axis . This local plane is usually defined as the plane tangent to the geodetic ellipsoid at the exposure point . The north of the local plane (+Y) The axis is the tangent of the local longitude on the ellipsoid , Point to the pole . The east of the local plane (+X) The axis is the tangent of the geodetic ellipsoid , Perpendicular to the local meridian . On the local plane (+Z) The axis is perpendicular to the earth ellipsoid , Perpendicular to the earth ellipsoid .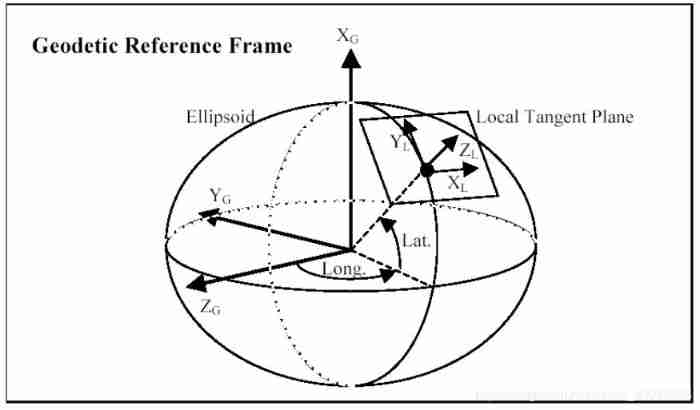
Can be applied Heading、Pitch、Roll Rotate to convert the original image to the correct position on the local tangent plane . Aligning the image axis with the plane axis is usually a very simple thing , May include exchange X and Y, Change the symbol on one or more angles , And apply the calibration offset . after , Apply translation 、 Pitch and roll rotation to map the original image to the local tangent plane .
camera / The coordinates of sensors are usually expressed in the so-called “ Centered on the earth ---- The earth is fixed ”(ECEF) Geodetic reference frame as benchmark .X、Y、Z The coordinates are measured from the center of the ellipsoid , Not on a plane . They can also use latitude 、 Longitude and height on ellipsoid . Traditionally , majority GPS/INS Applied to Geodetic applications , Among them, ellipsoid reference system is the first choice . However , For photogrammetric applications , We have to make a flat image , It can be used at any time in projection , And merge with other projection data . It is necessary for us to convert our local tangent plane into a mapping projection plane , Such as UTM etc. .
Now our image is on the course 、 pitch 、 The rolling is corrected , But mapped to a locally defined plane . The next step is to convert our local plane image into a world-based projection , Such as UTM. The local tangent plane is defined with reference to the geodetic ellipsoid . It is tangent to the ellipsoid at the exposure point .UTM Projection is formed by projecting the ellipsoid earth onto a cylinder . The cylinder is placed transversely on the ellipsoid , Tangent to the earth along the longitude and latitude line . The meridian of this tangent is called the central meridian . When we expand the cylinder to form a plan , The central meridian will remain along UTM+Y The vertical line of the axis . When you leave the central meridian , Other meridians will appear more and more upturned , Meet with the central meridian at the poles . The local longitude passing through the tangent point of the local plane usually does not coincide with the central longitude . This means that the local tangent plane X Axis and Y Shaft with UTM Projective X Axis and Y The axis will be different . In both systems ,Z The axis will be different everywhere , Unless the local tangent point is right on the central meridian .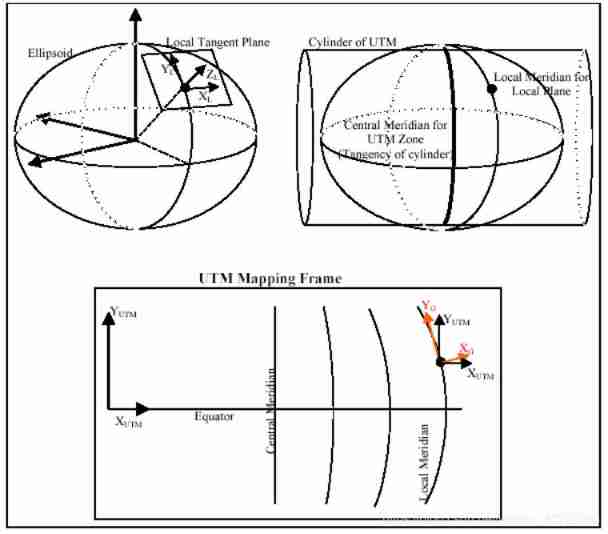
Omega, Phi, Kappa
Now say Omega、Phi、Kappa External posture of form . These three rotations are image reference system and plane 、 Projection mapping plane ( The most common is UTM) The transformation between . These three rotations are usually applied in sequence .
Omega - around X The rotation of the shaft
Phi - Around the Y Shaft rotation
Kappa - Around the Z Shaft rotation
These angles will produce a transformation with all of the above ( be based on Heading、Pitch、Roll) Same result , Directly map the original image to UTM Mapping plane . Because aerial photogrammetry mainly focuses on acquiring geographic coordinate system , So they usually only use Omega, Phi, Kappa.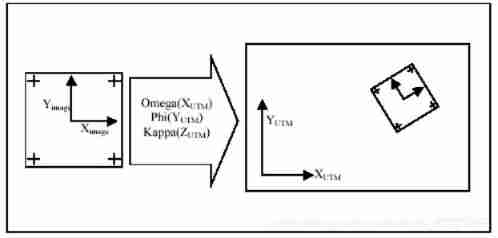
At present, some software can be converted to each other , hold Heading、Pitch、Roll Convert to Omega、Phi、Kappa. I am engaged in aerial photogrammetry , Commonly used empty three software, such as Inpho perhaps Photoscan And other software support two formats .
边栏推荐
- 安装OpenCV--conda建立虚拟环境并在jupyter中添加此环境的kernel
- Utf8 encoding
- Find the combination number acwing 887 Find combination number III
- Vant Weapp SwipeCell设置多个按钮
- Redis-02.Redis命令
- How to set the drop-down arrow in the spinner- How to set dropdown arrow in spinner?
- 5.Oracle-表空间
- Use ffmpeg to rotate, flip up and down, and flip horizontally
- AE tutorial - path growth animation
- La redirection de l'applet Wechat ne déclenche pas onload
猜你喜欢

背包问题 AcWing 9. 分组背包问题

International Open Source firmware Foundation (osff) organization

Game theory acwing 893 Set Nim game

Genesis builds a new generation of credit system

Gauss Cancellation acwing 884. Solution d'un système d'équations Xor linéaires par élimination gaussienne

Redis-01.初识Redis

Financial risk control practice -- feature derivation based on time series
ADG5412FBRUZ-RL7应用 双电源模拟开关和多路复用器IC
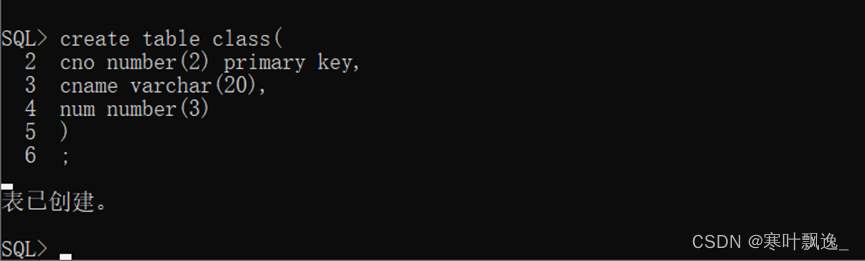
7. Oracle table structure

求组合数 AcWing 888. 求组合数 IV
随机推荐
Alibaba established the enterprise digital intelligence service company "Lingyang" to focus on enterprise digital growth
Chart. JS - Format Y axis - chart js - Formatting Y axis
Sum of three terms (construction)
Vant Weapp SwipeCell设置多个按钮
Client use of Argo CD installation
Idea debug failed
Financial risk control practice -- feature derivation based on time series
LSA Type Explanation - detailed explanation of lsa-2 (type II LSA network LSA) and lsa-3 (type III LSA network Summary LSA)
Redis-02.Redis命令
Series of how MySQL works (VIII) 14 figures explain the atomicity of MySQL transactions and the principle of undo logging
博弈论 AcWing 892. 台阶-Nim游戏
Modnet matting model reproduction
ollvm编译出现的问题纪录
Redis-02. Redis command
4.Oracle-重做日志文件管理
博弈论 AcWing 893. 集合-Nim游戏
C Primer Plus Chapter 15 (bit operation)
安装OpenCV--conda建立虚拟环境并在jupyter中添加此环境的kernel
NotImplementedError: Cannot convert a symbolic Tensor (yolo_boxes_0/meshgrid/Size_1:0) to a numpy ar
Suppose a bank's ATM machine, which allows users to deposit and withdraw money. Now there is 200 yuan in an account, and both user a and user B have the right to deposit and withdraw money from this a