Expected value (EV)

1. Expectations （Expected Value,EV）

Note source ：StatQuest series ：Expected Values, Main Ideas!!!

1.1 Expectation of discrete events

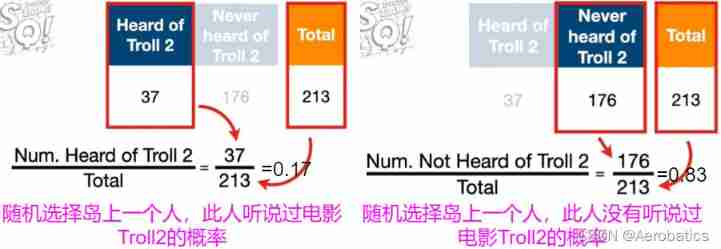
Start with a bet , The little man on the left of the picture below bets me , On the island StatLand Interview a resident of the island at random , If the resident has heard of movies Troll2, Then I lose to the villain 1dollar, If the resident has not heard of , Then I win 1dollar

Let's count the movies we've heard of on the island Troll2 The number of people 、 I haven't heard of movies Troll2 The number of people 、 The total number of

Let's calculate that we have heard of movies Troll2 The proportion of the number of people in the total number of people on the island 、 I haven't heard of movies Troll2 The proportion of the number of people in the total number of people on the island

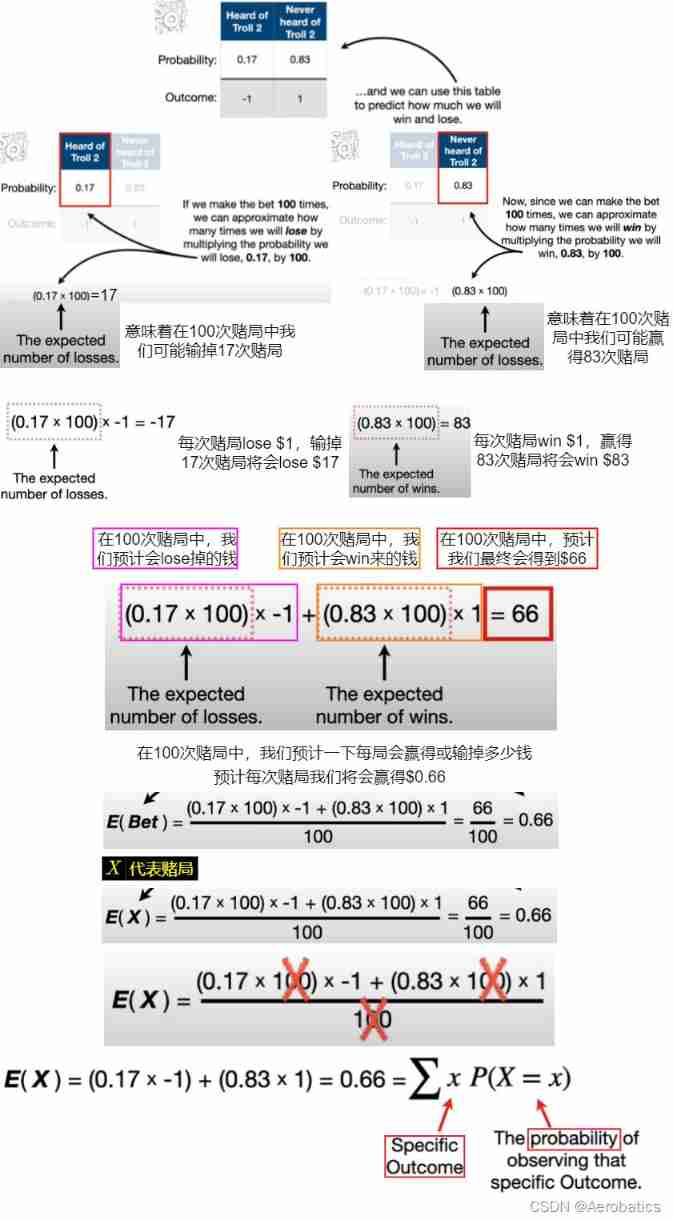
We will match the possible results of gambling with the statistical situation

No one wants to lose the bet , We fight with little people 100 Second gamble , We can predict how much money we can win or lose in the end

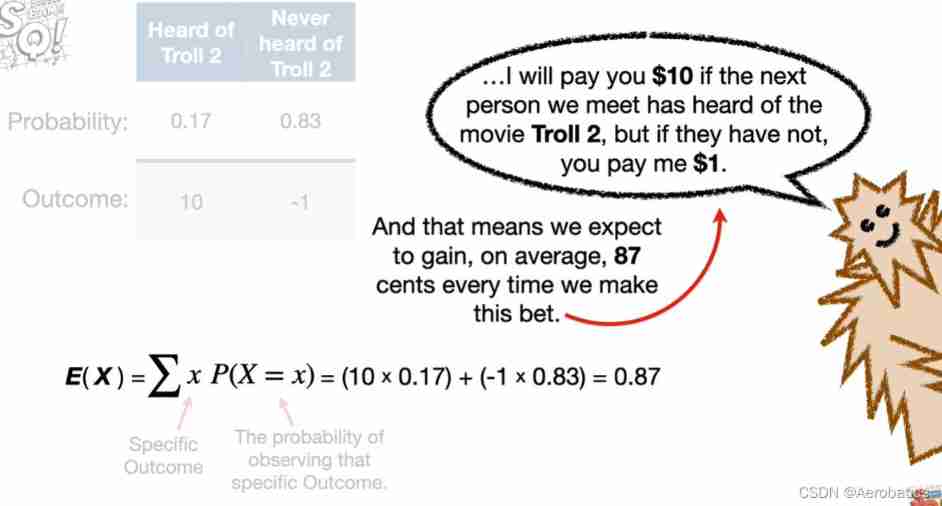
Suppose we bet more , Every time I win a bet, I will get 10dollar, If I lose, I will lose 1dollar
How much money can we win or lose in a lot of gambling ？ Please calculate the expected value

1.2 Expectations for continuous events

Note source ：Expected Values for Continuous Variables!!!

The little man walks on the road of the island , How long will it take to see someone ？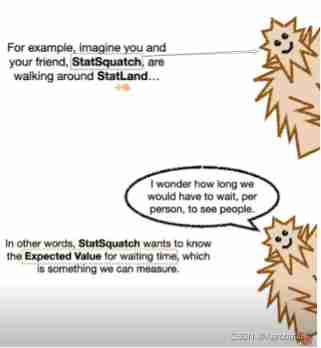

We use 10 Seconds as an interval , The results of an experiment are as follows ：
At the beginning, I met 7 personal , After that 10 Seconds later, I encountered 4 personal , After that 20 Seconds later, I encountered 2 people , After that 30 Seconds later, I encountered 2 people , After that 50 Seconds later, I encountered 2 people , After that 70 Seconds later, I encountered 2 people

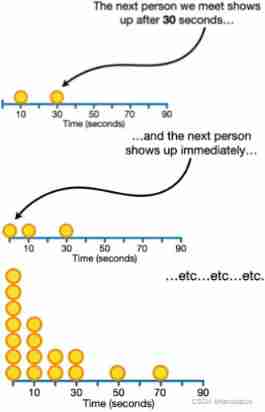
If you want to 5 Experiment at intervals of seconds ？ If you want to 2.5 Experiment at intervals of seconds ？ The smaller the time interval, the more comprehensive the experiment we get , Because you are at an interval of 10 Second experiment , If in the first place 5 Seconds met someone , You didn't count , Data loss , But we can't collect all the experimental data at intervals in our whole life , Because the time interval can be subdivided infinitely , From this we get continuous

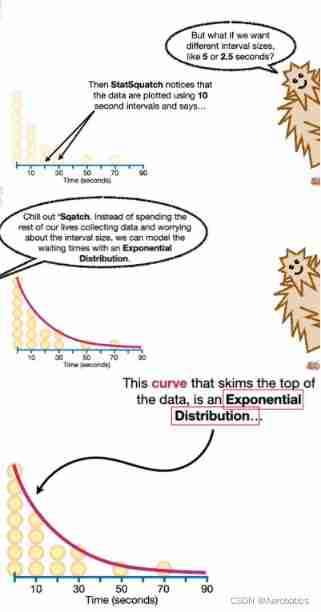
In this function of exponential distribution $\lambda$ Represents the number of people encountered per second

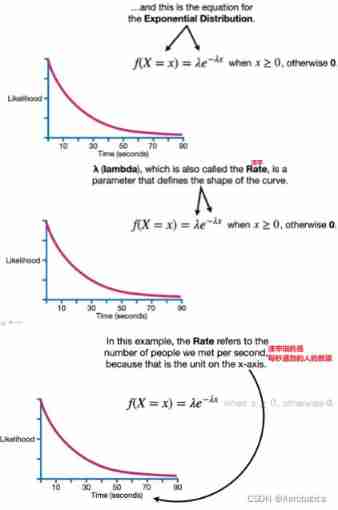
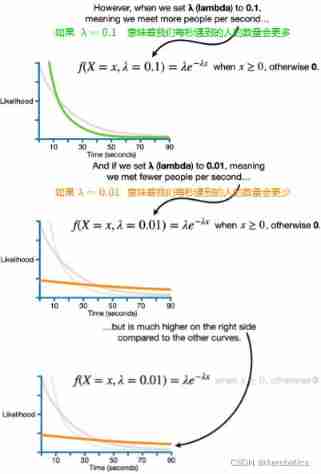
In the above experiment , When $\lambda =0.05$ when , The curve conforms to the current experimental data

We try to change $\lambda$ Value

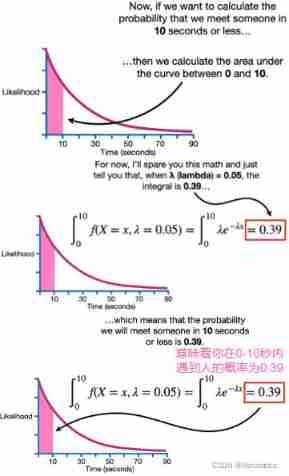
Let's calculate 0-10 The probability of meeting someone in seconds

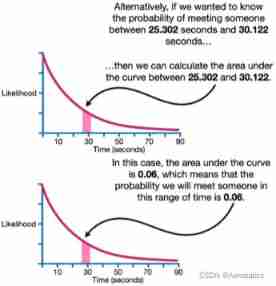
Allied , Let's calculate 25.302-30.122 The probability of meeting someone in seconds

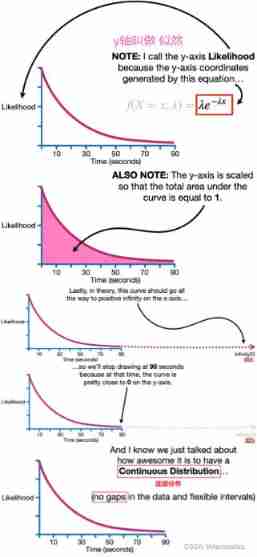
Notice about this exponential distribution
As mentioned below y Axis It's called likelihood （Likelihood）
The reasons are detailed in ：Maximum Likelihood for the Exponential Distribution, Clearly Explained!!!

Compare the expected value of discrete variables with the expected value of continuous variables

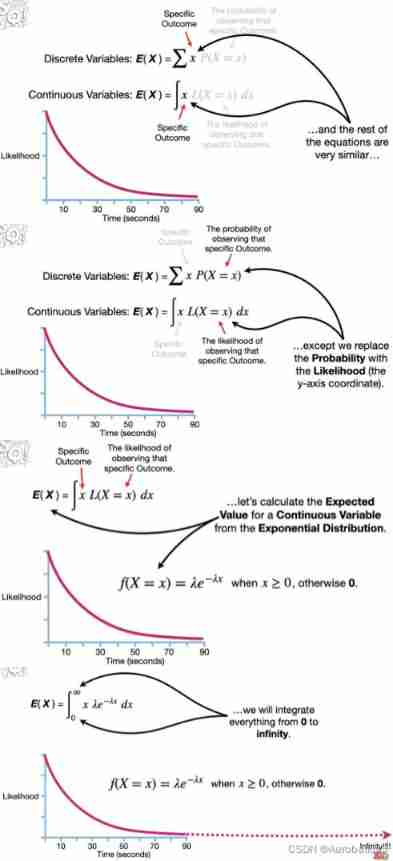
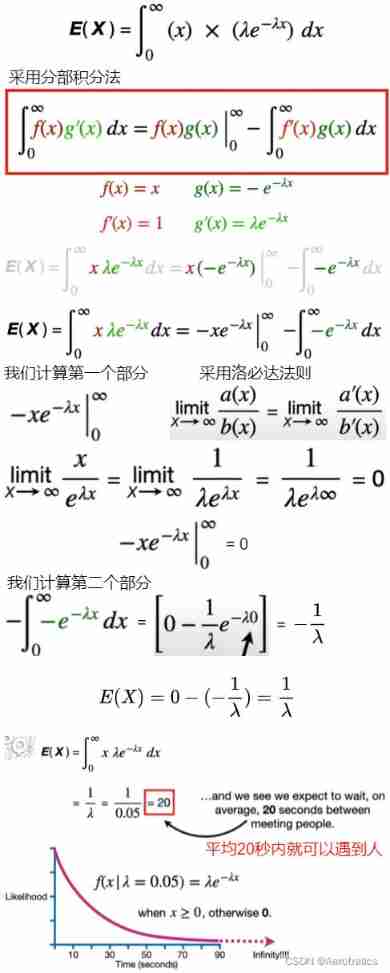
Next, we calculate the expected value of continuous variables