当前位置：网站首页>单调性约束与反单调性约束的区别 monotonicity and anti-monotonicity constraint

单调性约束与反单调性约束的区别 monotonicity and anti-monotonicity constraint

2022-07-07 08:39:00 【Xminyang】

▚ 01 Monotone Constraints 单调性约束

1.1 Definitions 定义

A constraint C is monotone if and only if for all itemsets S and S′:
if S ⊇ S′ and S violates C, then S′ violates C.

1.2 Key Points 要点

Monotone constraints possess the following property. If an itemset S violates a monotone constraint C, then any of its subsets also violates C. Equivalently, all supersets of an itemset satisfying a monotone constraint C also satisfy C (i.e., C is upward closed). By exploiting this property, monotone constraints can be used for reducing computation in frequent itemset mining with constraints. As frequent itemset mining with constraints aims to find frequent itemsets that satisfy the constraints, if an itemset S satisfies a monotone constraint C, no further constraint checking needs to be applied to any superset of S because all supersets of S are guaranteed to satisfy C. Examples of monotone constraints include min(S. Price) ≤ $30, which expresses that the minimum price of all items in an itemset S is at most $30. Note that, if the minimum price of all items in S is at most $30, adding more items to S would not increase its minimum price (i.e., supersets of S would also satisfy such a monotone constraint).

在这里插入图片描述

▚ 02 Anti-monotone Constraints 反单调性约束

2.1 Definitions 定义

【Anti-monotone Constraints | SpringerLink】

A constraint C is anti-monotone if and only if for all itemsets S and S′:
if S ⊇ S′and S satisfies C, then S′ satisfies C.

2.2 Key Points 要点

Anti-monotone constraints possess the following nice property. If an itemset S satisfies an anti-monotone constraint C, then all of its subsets also satisfy C (i.e., C is downward closed). Equivalently, any superset of an itemset violating an anti-monotone constraint C also violates C. By exploiting this property, anti-monotone constraints can be used for pruning in frequent itemset mining with constraints. As frequent itemset mining with constraints aims to find itemsets that are frequent and satisfy the constraints, if an itemset violates an anti-monotone constraint C, all its supersets (which would also violate C) can be pruned away and their frequencies do not need to be counted. Examples of anti-monotone constraints include min(S. Price) ≥ $20 (which expresses that the minimum price of all items in an itemset S is at least $20) and the usual frequency constraint support(S) ≥ minsup (i.e., frequency(S) ≥ minsup). For the former, if the minimum price of all items in S is less than $20, adding more items to S would not increase its minimum price (i.e., supersets of S would not satisfy such an anti-monotone constraint). For the latter, it is widely used in frequent itemset mining, with or without constraints. It states that (i) all subsets of a frequent itemset are frequent and (ii) any superset of an infrequent itemset is also infrequent. This is also known as the Apriori property.

在这里插入图片描述

▚ 03 Explanation 解释

假设：我们将S violates C作为事件A，S′ violates C作为事件B；则 S satisfies C为事件not A，then S′ satisfies C为事件not B.

此时，根据Monotone Constraints定义知(A → B)，也即(not B → not A)；
根据Anti-monotonicity Constraints定义知(not A → not B)，也即(B → A)；

因为(A → B)并不一定意味着(B → A)，所以这两者 (Monotone Constraints & Anti-monotonicity Constraints) 的声明是不同的。

在这里插入图片描述

▚ 04 Example 示例

For an example. Consider-  
C1 = Sum of elements is greater than 5  
C2 = Sum of elements is at most 5  
U(universe) = Set of non-negative real numbers

In case of C1,
If S violates C1, then S’ obviously violates C1 as well (S being a superset of S’)
Eg. S = {1, 2}, S’ = {2}
Hence C1 is monotonic.

In case of C2,
If S satisfies C2, then S’ obviously satisfies C2 as well (S being a superset of S’)
Eg. S = {1, 2}, S’ = {2}
Hence C2 is anti-monotonic.

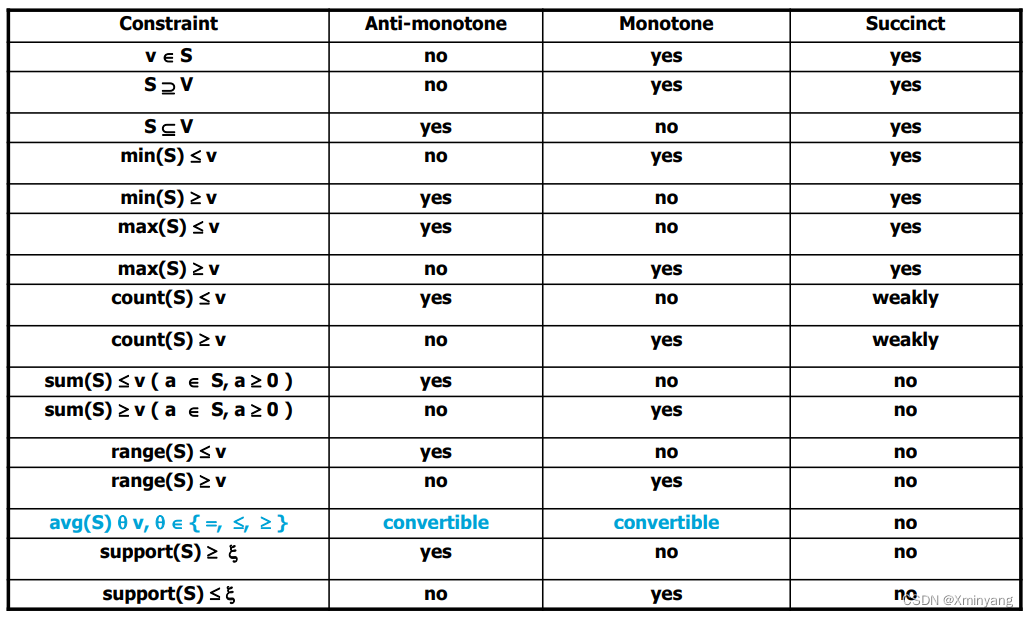
在这里插入图片描述