当前位置:网站首页>Obsidan之数学公式的输入
Obsidan之数学公式的输入
2022-07-07 05:23:00 【流岁金沙】
前言:
最近在学习专升本的高数,还想继续使用Obsidian作为笔记软件,但是苦于不知道数学公式怎么输入,于是有了这一篇文章
LaTex的语法
注意:这里的数学公式都要在$在这$,或者$$在这$$
先说下怎么换行
$$
\begin{aligned}a=b+c\\b=c-a\\c=a+b \end{aligned}
$$
a = b + c b = c − a c = a + b \begin{aligned}a=b+c\\b=c-a\\c=a+b \end{aligned} a=b+cb=c−ac=a+b
$$
\begin{matrix}已知y=\sqrt{x+3}&&(x>=0)\\求y的最大值是多少 \end{matrix}
$$
已 知 y = x + 3 ( x > = 0 ) 求 y 的 最 大 值 是 多 少 \begin{matrix}已知y=\sqrt{x+3}&&(x>=0)\\求y的最大值是多少 \end{matrix} 已知y=x+3求y的最大值是多少(x>=0)
$$
\begin{bmatrix}已知y=\sqrt{x+3}&&(x>=0)\\求y的最大值是多少 \end{bmatrix}
$$
[ 已 知 y = x + 3 ( x > = 0 ) 求 y 的 最 大 值 是 多 少 ] \begin{bmatrix}已知y=\sqrt{x+3}&&(x>=0)\\求y的最大值是多少 \end{bmatrix} [已知y=x+3求y的最大值是多少(x>=0)]
$$
\begin{Bmatrix}已知y=\sqrt{x+3}&&(x>=0)\\求y的最大值是多少 \end{Bmatrix}
$$
{ 已 知 y = x + 3 ( x > = 0 ) 求 y 的 最 大 值 是 多 少 } \begin{Bmatrix}已知y=\sqrt{x+3}&&(x>=0)\\求y的最大值是多少 \end{Bmatrix} { 已知y=x+3求y的最大值是多少(x>=0)}
$$
\begin{vmatrix}
0&1&2\\
3&4&5\\
6&7&8\\
\end{vmatrix}
$$
∣ 0 1 2 3 4 5 6 7 8 ∣ \begin{vmatrix} 0&1&2\\ 3&4&5\\ 6&7&8\\ \end{vmatrix} ∣∣∣∣∣∣036147258∣∣∣∣∣∣
$$
\begin{Vmatrix}
0&1&2\\
3&4&5\\
6&7&8\\
\end{Vmatrix}
$$
∥ 0 1 2 3 4 5 6 7 8 ∥ \begin{Vmatrix} 0&1&2\\ 3&4&5\\ 6&7&8\\ \end{Vmatrix} ∥∥∥∥∥∥036147258∥∥∥∥∥∥
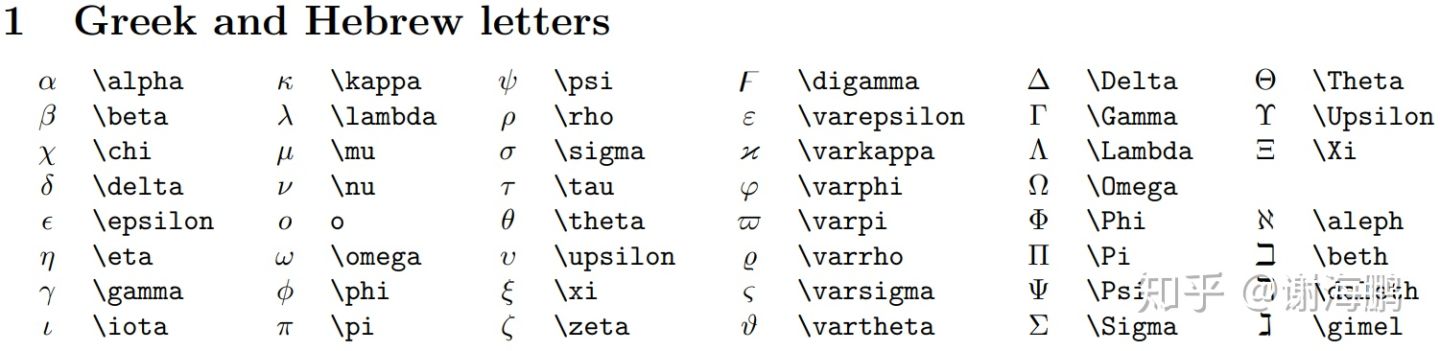
- 希腊字母
α \alpha α、 β \beta β、 χ \chi χ、 Δ \Delta Δ、 Γ \Gamma Γ、 Θ \Theta Θ之类的
- 一些数学结构

- 效果如下:
$\frac{123}{999}$、$\sqrt[n]{abc}$、$\frac{\sqrt{234}}{\sqrt[n]{abc}}$、$\underrightarrow{abc}$、$\overrightarrow{abc}$
123 999 \frac{123}{999} 999123、 a b c n \sqrt[n]{abc} nabc、 234 a b c n \frac{\sqrt{234}}{\sqrt[n]{abc}} nabc234、 a b c → \underrightarrow{abc} abc、 a b c → \overrightarrow{abc} abc
- 插入定界符

- 效果如下
$|$、$\|$、$\Uparrow$、$\{\}$
∣ | ∣、 ∥ \| ∥、 ⇑ \Uparrow ⇑、 { } \{\} { }
- 插入一些可变大小的符号

效果如下:
$\sum$、$\int$、$\oint$、$\iint$、$\bigcap\bigcup\bigoplus\bigotimes$
∑ \sum ∑、 ∫ \int ∫、 ∮ \oint ∮、 ∬ \iint ∬、 ⋂ ⋃ ⨁ ⨂ \bigcap\bigcup\bigoplus\bigotimes ⋂⋃⨁⨂
- 插入一些函数名称
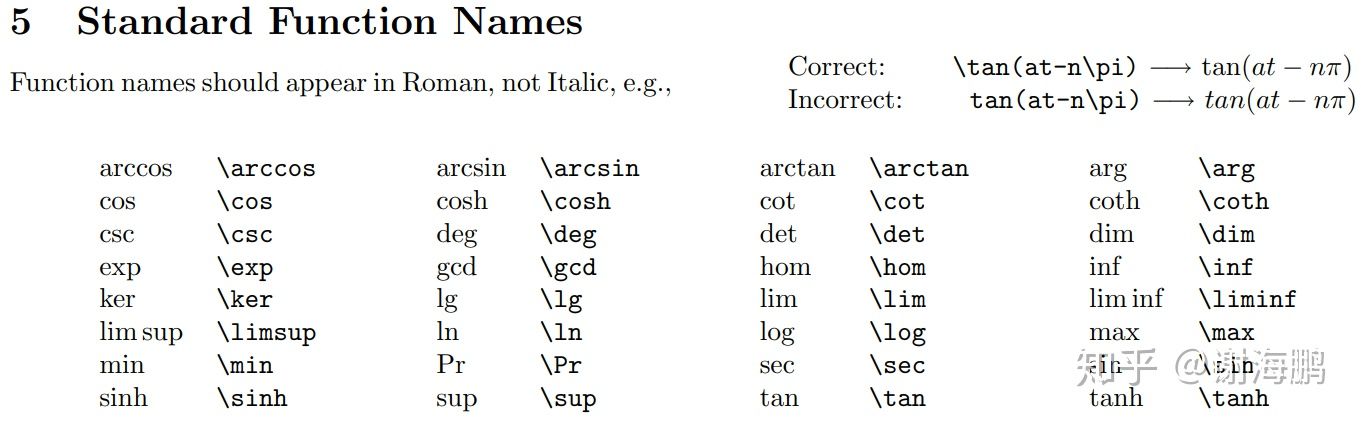
效果如下:
$\sin$、$\cos$、$\tan$、$\log$、 $\tan(at-n\pi)$
sin \sin sin、 cos \cos cos、 tan \tan tan、 log \log log、 tan ( a t − n π ) \tan(at-n\pi) tan(at−nπ)
- 关系运算符和二进制运算符

效果如下:
$\times$、$\ast$、$\div$、$\pm$、$\leq$、$\geq$、$\neq$、$\thickapprox$、$\sqsupset$、$\subset$、$\supseteq$、$\sqsupset$、$\sqsupseteq$、$\in$
× \times ×、 ∗ \ast ∗、 ÷ \div ÷、 ± \pm ±、 ≤ \leq ≤、 ≥ \geq ≥、 ≠ \neq =、 ≈ \thickapprox ≈、 ⊐ \sqsupset ⊐、 ⊂ \subset ⊂、 ⊇ \supseteq ⊇、 ⊐ \sqsupset ⊐、 ⊒ \sqsupseteq ⊒、 ∈ \in ∈
- 插入箭头符号

效果如下:
$\leftarrow$、$\Leftarrow$、$\nLeftarrow$、$\rightleftarrows$
← \leftarrow ←、 ⇐ \Leftarrow ⇐、 ⇍ \nLeftarrow ⇍、 ⇄ \rightleftarrows ⇄
- 其他符号
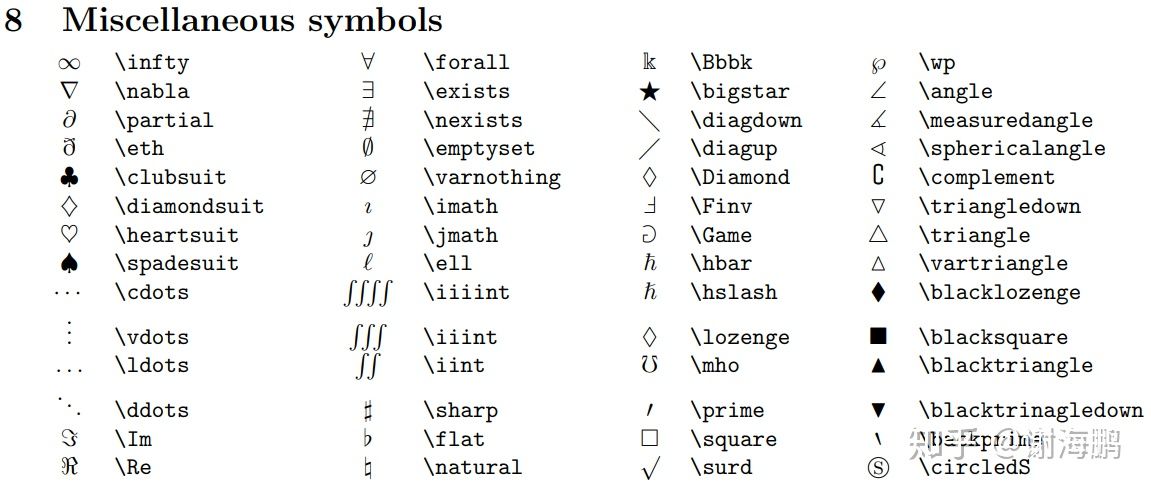
- 效果如下
$\infty$、$\angle$、$\int$、$\triangle$、$\square$
∞ \infty ∞、 ∠ \angle ∠、 ∫ \int ∫、 △ \triangle △、 □ \square □
- 插入上下标
用^表示上标,用_表示下标记
效果如下:
sin 2 ( θ ) + cos 2 ( θ ) = 1 \sin^2(\theta) + \cos^2(\theta) = 1 sin2(θ)+cos2(θ)=1
∑ n = 1 ∞ k \sum_{n=1}^\infty k n=1∑∞k
∫ a b f ( x ) d x \int_a^bf(x)\,dx ∫abf(x)dx
lim x → ∞ exp ( − x ) = 0 \lim\limits_{x\to\infty}\exp(-x) = 0 x→∞limexp(−x)=0
注意:
\,在积分里的作用是为了增加些许间距,\!会减少一些间距。输出分段函数
用\begin{cases}和\end{cases}来构造分段函数,中间则用\\来分段
f ( x ) = { 2 x , x > 0 3 x , x ≤ 0 f(x) = \begin{cases} 2x,\,\,x>0\\ 3x,\,\,x\le0\\ \end{cases} f(x)={ 2x,x>03x,x≤0
- 一些常见的数学公式
$$
f'(x) = x^2 + x
$$
f ′ ( x ) = x 2 + x f'(x) = x^2 + x f′(x)=x2+x
$$
\lim_{x\to0}\frac{9x^5+7x^3}{x^2+6x^8}
$$
lim x → 0 9 x 5 + 7 x 3 x 2 + 6 x 8 \lim_{x\to0}\frac{9x^5+7x^3}{x^2+6x^8} x→0limx2+6x89x5+7x3
$$
\int_a^b f(x)\,dx
$$
∫ a b f ( x ) d x \int_a^b f(x)\,dx ∫abf(x)dx
$$
\int_0^{+\infty}f(x)\,dx
$$
∫ 0 + ∞ f ( x ) d x \int_0^{+\infty}f(x)\,dx ∫0+∞f(x)dx
$$
\int_{x^2+y^2\leq R^2} \,f(x,y)\,dx\,dy = \int_{\theta=0}^{2\pi}\int_{r=0}^R \,f(r\cos\theta,r\sin\theta)\,r\,dr\,d\theta
$$
∫ x 2 + y 2 ≤ R 2 f ( x , y ) d x d y = ∫ θ = 0 2 π ∫ r = 0 R f ( r cos θ , r sin θ ) r d r d θ \int_{x^2+y^2\leq R^2} \,f(x,y)\,dx\,dy = \int_{\theta=0}^{2\pi}\int_{r=0}^R \,f(r\cos\theta,r\sin\theta)\,r\,dr\,d\theta ∫x2+y2≤R2f(x,y)dxdy=∫θ=02π∫r=0Rf(rcosθ,rsinθ)rdrdθ
$$
\int\!\!\!\int_D f(x,y)dxdy
$$
∫ ∫ D f ( x , y ) d x d y \int\!\!\!\int_D f(x,y)dxdy ∫∫Df(x,y)dxdy
参考:
https://zhuanlan.zhihu.com/p/158156773
边栏推荐
- 数据库实时同步利器——CDC(变化数据捕获技术)
- [quick start of Digital IC Verification] 10. Verilog RTL design must know FIFO
- Use of JMeter
- Offer harvester: add and sum two long string numbers (classic interview algorithm question)
- XCiT学习笔记
- Complex network modeling (III)
- 解析创新教育体系中的创客教育
- Practice of implementing cloud native Devops based on rainbow library app
- Openvscode cloud ide joins rainbow integrated development system
- Qinglong panel -- finishing usable scripts
猜你喜欢

Give full play to the wide practicality of maker education space

Notes on PHP penetration test topics
Openvscode cloud ide joins rainbow integrated development system
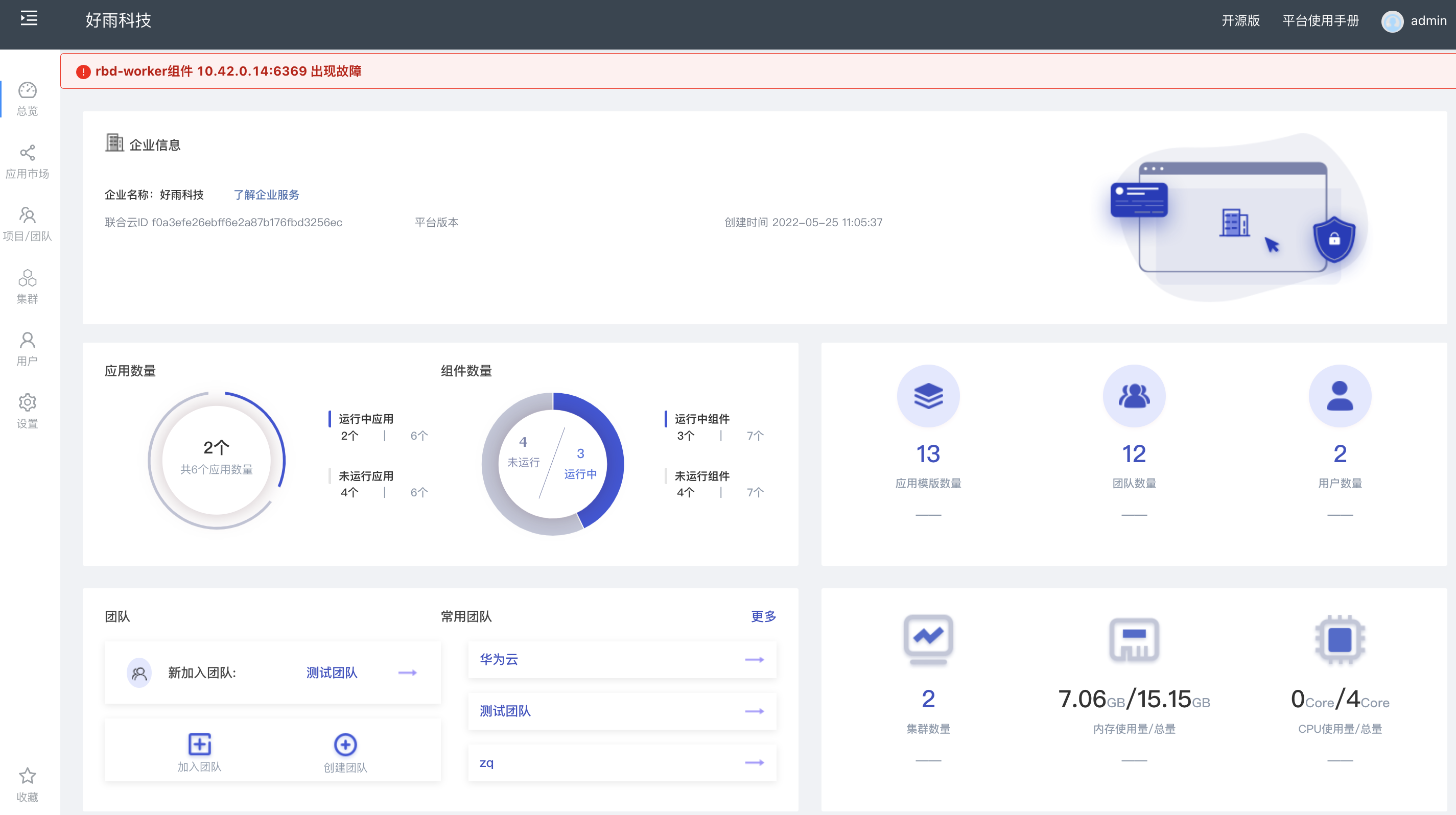
Rainbond 5.7.1 支持对接多家公有云和集群异常报警

CCTV is so warm-hearted that it teaches you to write HR's favorite resume hand in hand
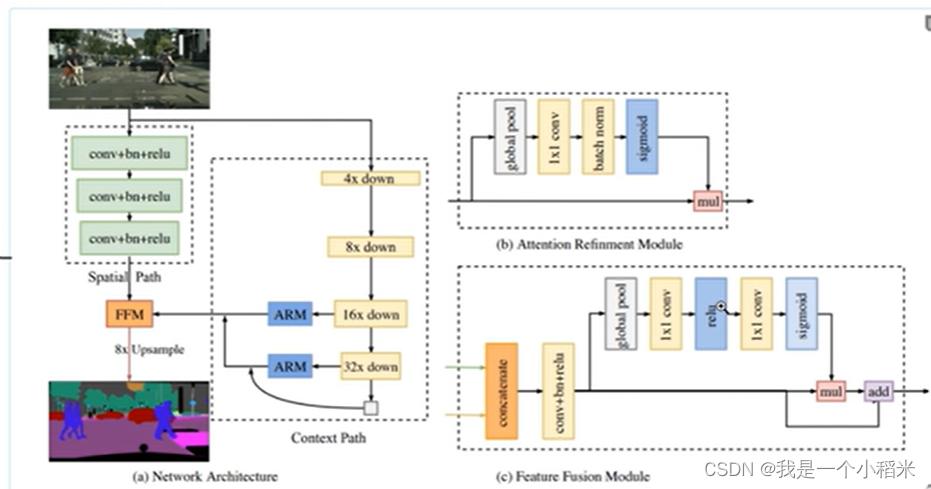
Bisenet features

Quick analysis of Intranet penetration helps the foreign trade management industry cope with a variety of challenges

BiSeNet的特點

Analyzing the influence of robot science and technology development concept on Social Research
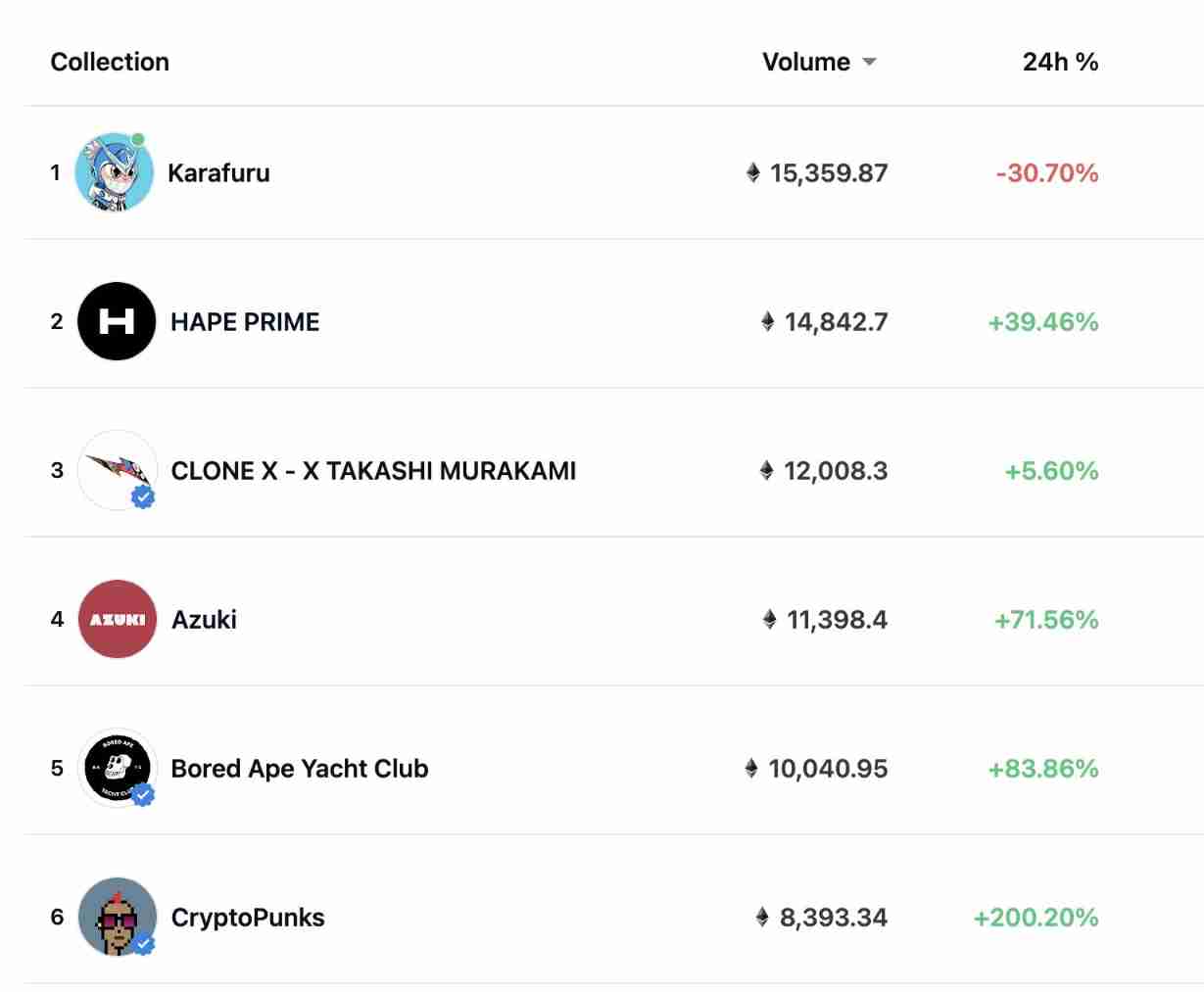
Wang Zijian: is the NFT of Tencent magic core worth buying?
随机推荐
Complex network modeling (III)
漏洞复现-easy_tornado
利用 Helm 在各类 Kubernetes 中安装 Rainbond
Domain specific language / DSL in kotlin
Openvscode cloud ide joins rainbow integrated development system
Zcmu--1396: queue problem (2)
Explore creativity in steam art design
Leetcode simple question: find the K beauty value of a number
Interactive book delivery - signed version of Oracle DBA work notes
Ebpf cilium practice (1) - team based network isolation
Battery and motor technology have received great attention, but electric control technology is rarely mentioned?
Pytoch (VI) -- model tuning tricks
Function extension, attribute extension and non empty type extension in kotlin
JS cross browser parsing XML application
GFS分布式文件系统
Rainbow 5.7.1 supports docking with multiple public clouds and clusters for abnormal alarms
饥荒云服管理脚本
Qinglong panel -- Huahua reading
复杂网络建模(二)
MES系統,是企業生產的必要選擇