当前位置:网站首页>ArabellaCPC 2019(补题)
ArabellaCPC 2019(补题)
2022-07-06 03:05:00 【WDLieqi】
D. Meeting Bahosain
题目大意
a,b两个数组,其中b数组里面的元素都是不同的,问我们是不是可以在a里面选一个元素,用b里面的元素对其进行无限次的加减,最后让a数组元素相同。比赛时根本不懂题目意思,裂开。
解题思路
要让所有元素相同,那么a[i] - a[j] (i, j 为任意的数)就要等于x1 * b1 + x2 * b2 + x3 * b3 + … + xn * bn。这里就要引入一个定理:a * x + b * y = gcd(a, b) 一定有解。代入进去我们可以得出 x1 * b1 + x2 * b2 + x3 * b3 + … + xn * bn = gcd(b1, b2, b3, …, bn)一定有解,这里因为乘数是自己取得,所以可得出 a[ i ] - a[ j ] = x * (gcd(b1, b2, …, bn)),x是任意常数。
最后就得出结论了,只要所有的a[ i ] - a[ j ]整除gcd(b1, b2, …, bn)就可以了。
代码
#include <bits/stdc++.h>
using namespace std;
#define IOS \
ios::sync_with_stdio(false); \
cin.tie(0); \
cout.tie(0);
//#define For(i, k, n) for (int i = k; i < n; i ++)
//#define FOR(i, k, n) for (int i = k; i <= n; i ++)
#define P acos(-1);
typedef long long LL;
typedef unsigned long long ULL;
#define PII pair<int, int>
#define PDD pair<double, double>
#define Pll pair<LL, LL>
typedef unsigned long long Uint;
const double eps = 1e-7;
const int M = 1e6 + 10, mod = 1000000007;
const int inf = 0x3f3f3f3f;
int dx[4] = {0, 1, -1, 0}, dy[4] = {1, 0, 0, -1};
int lowbit(int x) { return (x & -x); };
// 以上可忽略
const int N = 1e6 + 10;
int a[N], b[N];
void solve()
{
int n, k;
cin >> n >> k;
for (int i = 0; i < n; i ++)
cin >> a[i];
int res = 0;
for (int i = 0; i < n; i ++)
cin >> b[i], res = (res == 0) ? b[i] : __gcd(res, b[i]);
for (int i = 1; i < n; i ++)
{
if ((a[i] - a[i - 1]) % res != 0)
{
puts("No");
return;
}
}
puts("Yes");
}
int main()
{
IOS
int T = 1;
//cin >> T;
//scanf("%d", &T);
while (T--)
{
solve();
}
return 0;
}
I. Bashar and Hamada
题目大意
k(2 <= k <= n) 长度的数字问相互之间的差值的绝对值的最大值是多少。
解题思路
首先我们应该明白顺序是不影响大小的。然后再找一个规律:
假定一组数据 {1,2,3,4,5,7},开始ans=0;
开始:ans+= |1-7|
插入5: ans+=|5-1|+|5-7|=|1-7|
插入2: ans+=|2-1|+|2-7|+|2-5|==|1-7|+|2-5|
插入4: ans+=|4-1|+|4-7|+|4-5|+|4-2|==|1-7|+|2-5|
插入3: ans+=|3-1|+|3-7|+|3-5|+|3-2|+|3-4|==|1-7|+|2-5|+|3-4|
这样列出来就很明显了。奇数次插入,要加的数保存不变。
可以看到每插入2个就增加一个组合,只要每次加入的组合为最大,就能保证答案最大。
每次组合都选差最大的,排序后,就是左右各一个。
思路转载自这个博客:
https://www.cnblogs.com/studyshare777/p/12766707.html
代码
#include <bits/stdc++.h>
using namespace std;
#define IOS \
ios::sync_with_stdio(false); \
cin.tie(0); \
cout.tie(0);
//#define For(i, k, n) for (int i = k; i < n; i ++)
//#define FOR(i, k, n) for (int i = k; i <= n; i ++)
#define P acos(-1);
typedef long long LL;
typedef unsigned long long ULL;
#define PII pair<int, int>
#define PDD pair<double, double>
#define Pll pair<LL, LL>
typedef unsigned long long Uint;
const double eps = 1e-7;
const int N = 3e5 + 10, M = 1e6 + 10, mod = 1000000007;
const int inf = 0x3f3f3f3f;
int dx[4] = {0, 1, -1, 0}, dy[4] = {1, 0, 0, -1};
int lowbit(int x) { return (x & -x); };
LL f[N];
void solve()
{
LL n;
cin >> n;
for (int i = 1; i <= n; i ++)
cin >> f[i];
sort(f + 1, f + 1 + n);
LL ans = 0, tot = 0;
ans = f[n] - f[1];
tot = ans;
cout << ans << ' ';
int r = n - 1, l = 2;
for (int i = 1; i < n - 1; i ++)
{
if (!(i & 1))
tot += f[r --] - f[l ++];
ans += tot;
cout << ans << ' ';
}
}
int main()
{
IOS
int T = 1;
//cin >> T;
//scanf("%d", &T);
while (T--)
{
solve();
}
return 0;
}
边栏推荐
- tcpdump: no suitable device found
- Solution: attributeerror: 'STR' object has no attribute 'decode‘
- Introduction to robotframework (II) app startup of appui automation
- What are the principles of software design (OCP)
- 【若依(ruoyi)】启用迷你导航栏
- 深度解析链动2+1模式,颠覆传统卖货思维?
- 八道超经典指针面试题(三千字详解)
- Descriptor implements ORM model
- 3857墨卡托坐标系转换为4326 (WGS84)经纬度坐标
- Résumé des méthodes de reconnaissance des caractères ocr
猜你喜欢

js 正则过滤和增加富文本中图片前缀
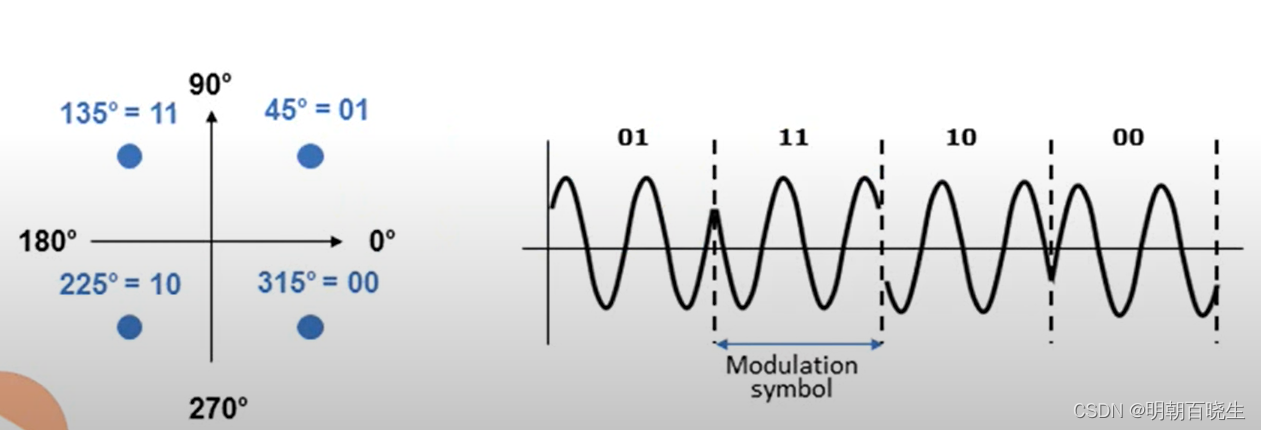
NR modulation 1
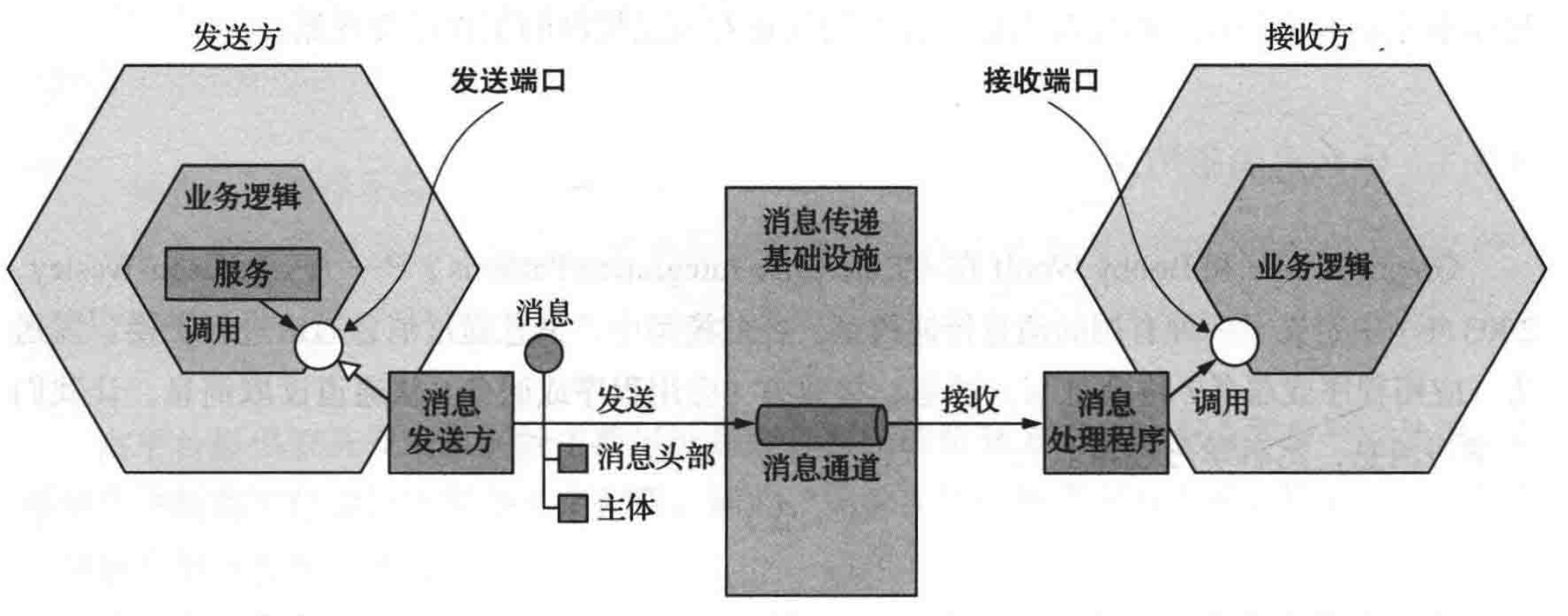
Communication between microservices

I sorted out a classic interview question for my job hopping friends
![BUUCTF刷题笔记——[极客大挑战 2019]EasySQL 1](/img/37/c38a933ce7fa5d2b8fa597965ffcb2.png)
BUUCTF刷题笔记——[极客大挑战 2019]EasySQL 1
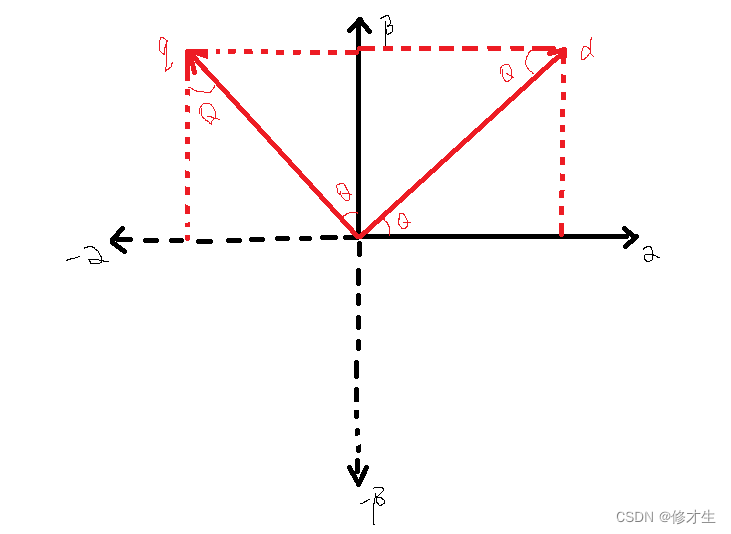
电机控制反Park变换和反Clarke变换公式推导
【Unity3D】GUI控件
适合程序员学习的国外网站推荐
Master data management theory and Practice
![[Chongqing Guangdong education] higher mathematics I reference materials of Southwest Petroleum University](/img/0f/520242492524522c887b6576463566.jpg)
[Chongqing Guangdong education] higher mathematics I reference materials of Southwest Petroleum University
随机推荐
Solve 9 with C language × 9 Sudoku (personal test available) (thinking analysis)
这些不太会
Referenceerror: primordials is not defined error resolution
C语言sizeof和strlen的区别
js 正则过滤和增加富文本中图片前缀
Descriptor implements ORM model
1003 emergency (25 points), "DIJ deformation"
【若依(ruoyi)】ztree 自定义图标(iconSkin 属性)
#PAT#day10
OCR文字識別方法綜述
[matlab] access of variables and files
【 kubernets series】 a Literature Study on the Safe exposure Applications of kubernets Service
Selenium share
My C language learning record (blue bridge) -- on the pointer
Briefly describe the implementation principle of redis cluster
2022工作中遇到的问题四
Codeworks 5 questions per day (1700 average) - day 6
I sorted out a classic interview question for my job hopping friends
PMP practice once a day | don't get lost in the exam -7.5
jsscript