当前位置:网站首页>Mathematical model Lotka Volterra
Mathematical model Lotka Volterra
2022-07-05 23:51:00 【jeff one】
mathematical model Lotka-Volterra
origin :
20 century 40 years ,Lotka(1925) and Volterra(1926) Laid a theoretical foundation for interspecific competition , The interspecific competition equation proposed by them has a significant impact on the development of modern ecological theory .
Content :
Lotka-Volterra Model (Lotka-Volterra Interspecific competition model ) yes logistic Model ( Growth retardation model ) Extension of . Now set the following parameters :
N1、N2: Are the population numbers of the two species
K1、K2: The environmental capacity of the two species
r1、r2 : Are the population growth rates of the two species
According to the logistic model, there are the following relationships :
among :N/K It can be understood as the space that has been used ( be called “ Space item used ”), be (1-N/K) It can be understood as unused space ( be called “ Unused space items ”)
When two species compete or use the same space ,“ Space item used ” You should also add N2 The occupation of space by the population . be :

among ,α: species 2 For species 1 Competition coefficient , each N2 The space occupied by individuals is equivalent to α individual N1 Space occupied by individuals .
Then there are ,β: species 1 For species 2 Competition coefficient , each N1 The space occupied by individuals is equivalent to β individual N2 Space occupied by individuals . There is another :

As we know :
When species N1 population ( species 1) The environmental capacity of is K1 when ,N1 The inhibitory effect of each individual in the population on the growth of its own population is 1/K1; Empathy ,N2 The inhibitory effect of each individual in the population on the growth of its own population is 1/K2.
in addition , from (1)、(2) Two equations and α、β From the definition of :
N2 Each individual in the population pairs N1 The effect of population is :α/K1
N1 Each individual in the population pairs N2 The effect of population is :β/K2
therefore , When species 2 Can inhibit species 1 when , It can be said that , species 2 For species 1 Influence > species 2 The impact on oneself , namely α/K1 > 1/K2.
边栏推荐
猜你喜欢

PADS ROUTER 使用技巧小记
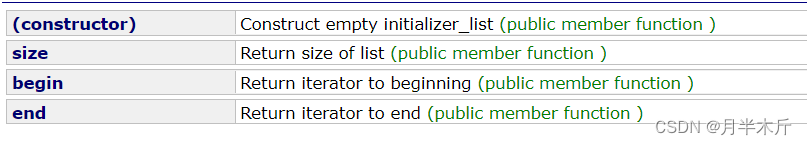
Initialize your vector & initializer with a list_ List introduction

保研笔记四 软件工程与计算卷二(8-12章)
CAS and synchronized knowledge

Rasa 3. X learning series -rasa x Community Edition (Free Edition) changes
20.移植Freetype字体库
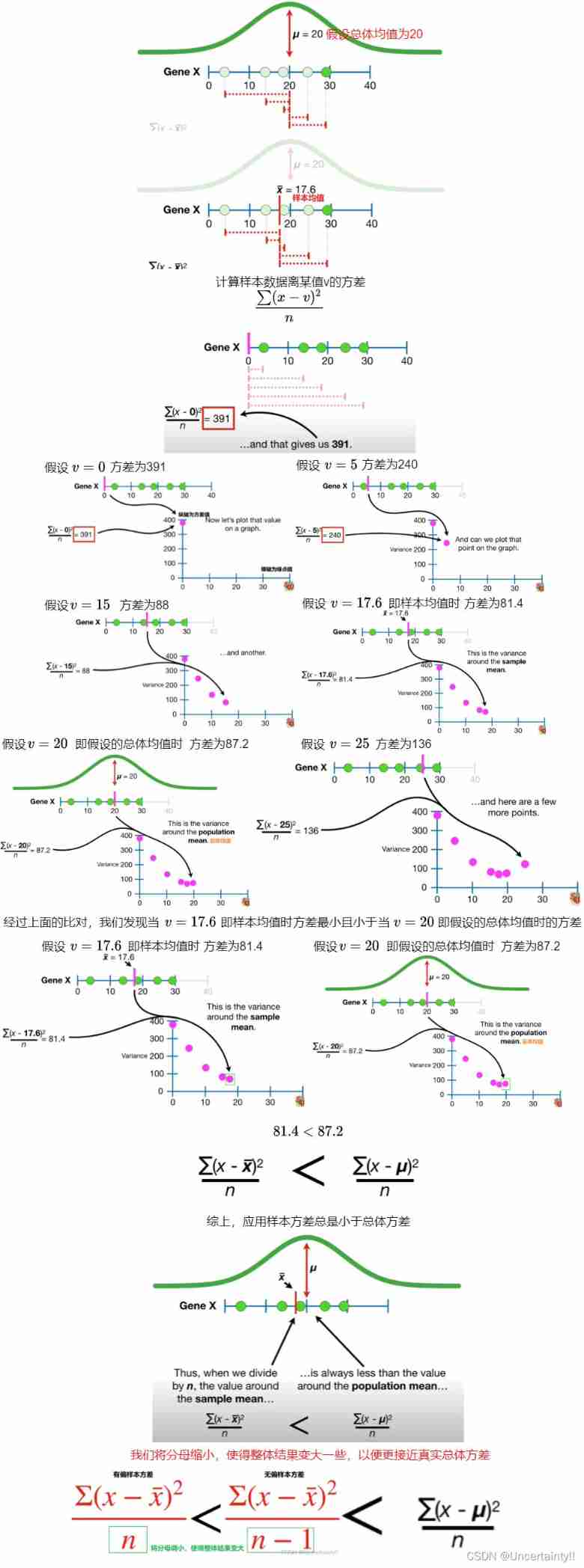
Biased sample variance, unbiased sample variance
Redis high availability - master-slave replication, sentinel mode, cluster
4点告诉你实时聊天与聊天机器人组合的优势
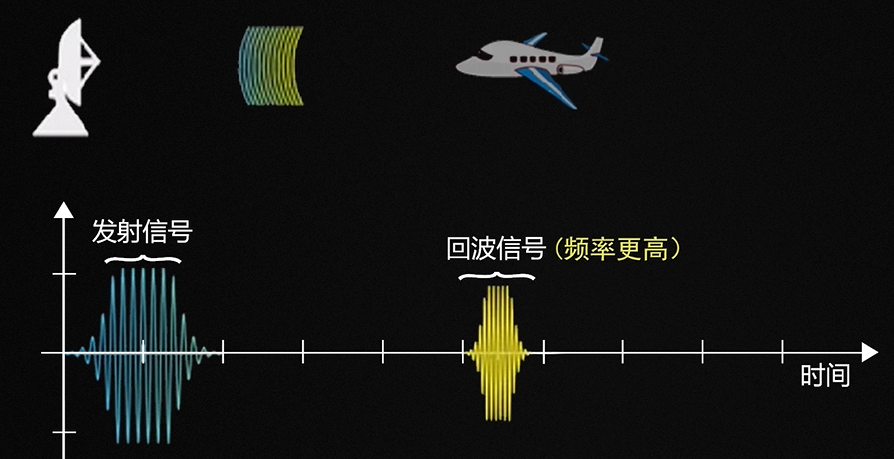
多普勒效應(多普勒頻移)
随机推荐
[Luogu cf487e] tours (square tree) (tree chain dissection) (line segment tree)
VBA fast switching sheet
Rasa 3. X learning series -rasa 3.2.1 new release
【GYM 102832H】【模板】Combination Lock(二分图博弈)
Golang code checking tool
Spire Office 7.5.4 for NET
15 MySQL-存储过程与函数
Introduction to JVM
20220703 周赛:知道秘密的人数-动规(题解)
20220703 week race: number of people who know the secret - dynamic rules (problem solution)
传输层协议------UDP协议
idea 连接mysql ,直接贴配置文件的url 比较方便
TS type declaration
《牛客刷verilog》Part III Verilog企业真题
【LeetCode】5. Valid palindrome
Which side projects can be achieved? Is it difficult for we media to earn more than 10000 a month?
妙才周刊 - 8
GFS distributed file system
多普勒效應(多普勒頻移)
Redis高可用——主从复制、哨兵模式、集群