Statistics, 8th Edition, Jia Junping, Chapter VIII, summary of knowledge points of hypothesis test and answers to exercises after class

Catalog

 

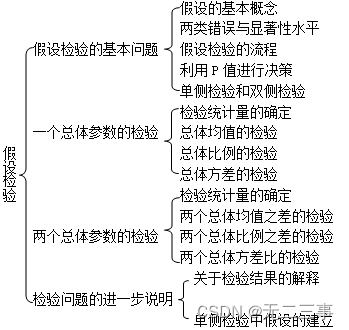
One 、 Examination site induction

Two 、 After-school exercises

---

One 、 Examination site induction

Two 、 After-school exercises

1 It is known that the carbon content of an ironmaking plant follows a normal distribution N（4.55,0.1082）, Now we have measured 9 Hot metal , Its average carbon content is 4.484. If the estimated variance does not change , Can we think that the average carbon content of molten iron produced now is 4.55（α＝0.05）？

Explain ： Make assumptions ：
H0：μ＝4.55;H1：μ≠4.55
This is a bilateral test , And the variance is known , Test statistics Z The value is ：

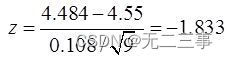
and z0.025＝1.96＞|－1.833|, Therefore, the original hypothesis cannot be rejected , It can be considered that the average carbon content of molten iron produced now is 4.55.

2 There is an element , Its service life shall not be less than 700 Hours . Now we randomly select from a batch of such components 36 Pieces of , Its average life is measured to be 680 Hours . It is known that the service life of the element follows a normal distribution ,σ＝60 Hours , Try at the significance level 0.05 Determine whether this batch of components are qualified .

Explain ： Make assumptions ：
H0：μ≥700;H1：μ＜700
This is the left side inspection , And the variance is known , Test statistic z by ：

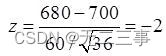
and －z0.05＝－1.645＞－2, Therefore, the original hypothesis is rejected , That is, at the significance level 0.05 The next batch of components is unqualified .

3 The general production level of wheat in a certain area is per mu 250 kg , The standard deviation is 30 kg . Now a kind of chemical fertilizer is used for experiment , from 25 Sampling of plots , The average output is 270 kg . Whether this chemical fertilizer can significantly increase the yield of wheat （α＝0.05）？

Explain ： Make assumptions ：
H0：μ≤250;H1：μ＞250

  This is the right test , And the variance is known , Test statistics z The value is ：

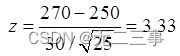
and z0.05＝1.645＜3.33, Therefore, the original hypothesis is rejected , That is, this chemical fertilizer has significantly increased the yield of wheat .

4 Sugar factories use automatic balers to pack , The standard weight of each package is 100 kg . After starting work every day, it is necessary to check whether the packer works normally . It was measured after starting work on a certain day 9 Package weight （ Company ： kg ） as follows ：99.3　98.7　100.5　101.2　98.3　99.7　99.5　102.1　100.5, It is known that the weight of each package follows a normal distribution , Try to check whether the packer works normally on that day （α＝0.05）？

Explain ： Make assumptions ：
H0：μ＝100;H1：μ≠100

From the sample data ：

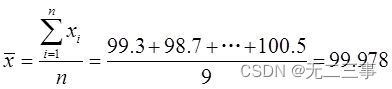
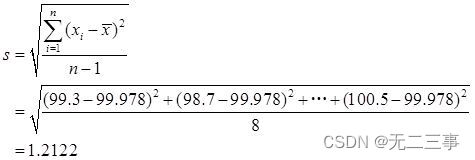
This is a bilateral test , And the variance is unknown , Another small sample , Therefore adopt t statistic , The value of the test statistic is ：

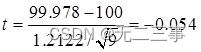
and t0.025（8）＝2.306＞|－0.054|, Therefore, the original assumption is not rejected , That is, the packer works normally on that day .

5 A certain mass-produced packaged food shall not be less than 250 g . This is a random sample from a batch of this food 50 bag , Found to have 6 Bag below 250 g . If the specified percentage of non-compliance exceeds 5% You can't leave the factory , Ask whether this batch of food can leave the factory （α＝0.05）？

Explain ： Make assumptions ：
H0：π≤5%;H1：π＞5%
p＝6/50＝12%,

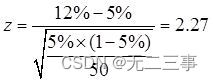
This is a one-sided test , When α＝0.05 when , Yes z0.05＝1.645＜2.27, Therefore, the original hypothesis is rejected , That is, this batch of food cannot leave the factory .

6 A manufacturer claims in an advertisement , Under normal conditions, the running distance of the automobile tires produced by this factory exceeds the current average 25000 km . For one by 15 A random sample of tires was tested , The mean and standard deviation of the samples are obtained as 27000 Km and 5000 km . Assume that the tire life follows a normal distribution , Ask whether the content claimed in the manufacturer's advertisement is true （α＝0.05）？

Explain ： Make assumptions ：
H0：μ≤25000;H1：μ＞25000
This is a one-sided test , And the variance is unknown , Another small sample , Therefore adopt t statistic , The value of the test statistic is ：

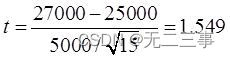
and t0.05（14）＝1.761＞1.549, Therefore, the original hypothesis cannot be rejected , That is, the advertisement of the manufacturer is not true .

7 The life of an electronic component x（ Company ： Hours ） It follows a normal distribution . Now measured 16 The service life of only components is as follows ：

Ask whether there is reason to think that the average life of components is significantly greater than 225 Hours （α＝0.05）？

Explain ： Make assumptions ：
H0：μ≤225;H1：μ＞225
From the sample data ：

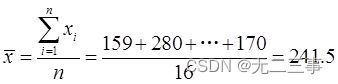
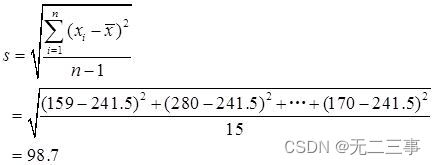
This is a one-sided test , And the variance is unknown , Another small sample , Therefore adopt t statistic , The value of the test statistic is ：

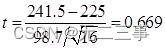
and t0.05（15）＝1.753＞0.669, Therefore, the original hypothesis cannot be rejected , There is no reason to think that the average life of components is significantly greater than 225 Hours .

8 Random sampling 9 A unit of , The measured results are ：

85　59　66　81　35　57　55　63　66, With α＝0.05 The following assumptions are tested by the significance level of ：H0：σ2≤100;H1：σ2＞100.

Explain ： From the sample data ：

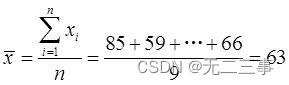
This is a one-sided test , And the mean value is unknown , Test statistics χ2 The value is ：

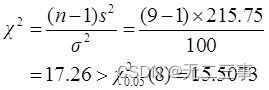
So reject the original assumption .

9A,B The two factories produce the same materials . It is known that its compressive strength follows a normal distribution , And σA2＝632,σB2＝572. from A Randomly selected from the materials produced by the factory 81 Samples , Measured xA＝1070kg/cm2; from B Randomly selected from the materials produced by the factory 64 Samples , Measured xB＝1020kg/cm2. According to the above findings , Can you think A,B The average compressive strength of the materials produced by the two plants is the same （α＝0.05）？ Explain ： Make assumptions ：
H0：μA－μB＝0;H1：μA－μB≠0
This is a bilateral test , also σA2、σB2 It is known that , The value of the test statistic is ：

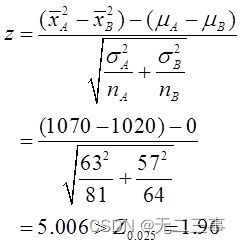
So reject the original assumption . That is, you can't think A、B The average compressive strength of the materials produced by the two plants is the same .

10 There are different ways to assemble a part , The concern is which method is more efficient . Labor efficiency can be reflected by average assembly time . Now from different assembly methods 12 Products , Record the respective assembly time （ Company ： minute ） as follows ：

The two populations are normal populations , And the variance is the same . Ask whether there is a significant difference in assembly time between the two methods （α＝0.05）？

Explain ： Make assumptions ：
H0：μA－μB＝0;H1：μA－μB≠0
From the sample data ：

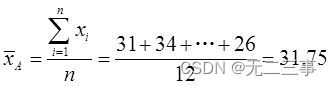
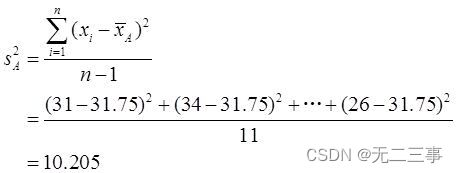
The same can be ：xB＝28.67,sB2＝6.061.

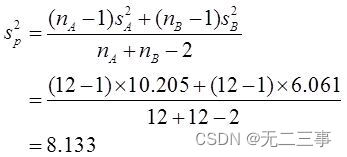
This is a bilateral test , And the two populations are normal populations , The variance is the same , Test statistics t by ：

So reject the original assumption , That is, the assembly time of these two methods is significantly different .

11 A survey of 339 name 50 People over the age of , among 205 Among smokers 43 One has chronic tracheitis , stay 134 Among the non-smokers 13 People suffer from chronic tracheitis . Can the survey data support “ Smokers are prone to chronic tracheitis ” This view （α＝0.05）？

  Explain ： Make assumptions ：
H0：π1－π2≤0（ Smokers are not prone to chronic tracheitis ）
H1：π1－π2＞0（ Smokers are prone to chronic tracheitis ）
This is a one-sided test , Test statistics z by ：

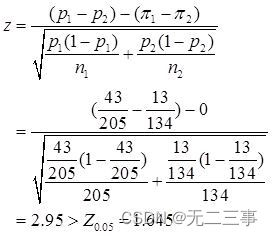
So reject the original assumption , That is, survey data support “ Smokers are prone to chronic tracheitis ” Point of view .

12 In order to control the loan scale , A commercial bank has an internal requirement , The average amount of each loan cannot exceed 60 Ten thousand yuan . With the development of economy , The loan scale tends to increase . The bank manager would like to know that under the same project conditions , Whether the average size of loans significantly exceeds 60 Ten thousand yuan , So one n＝144 A random sample of is drawn , Measured x＝68.1 Ten thousand yuan ,s＝45. stay α＝0.01 Under the significance level of P The value is tested .

Explain ： Make assumptions ：
H0：μ≤60;H1：μ＞60
This is a one-sided test , And it is a large sample , Test statistic z by ：

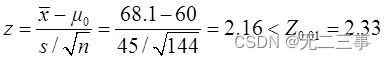
So we can't reject the original hypothesis , That is, the average size of loans did not significantly exceed 60 Ten thousand yuan .

13 There is a theory that taking aspirin can help reduce the incidence of heart disease , For verification , Researchers put those who volunteered to participate in the experiment 22000 People were randomly divided into two groups , One group took aspirin three times a week （ sample 1）, Another group took a placebo at the same time （ sample 2）. continued 3 Test after years , sample 1 There is 104 People suffer from heart disease , sample 2 There is 189 People suffer from heart disease . With α＝0.05 To test whether taking aspirin can reduce the incidence of heart disease .

Explain ： Make assumptions ：
H0：π1－π2≥0（ Taking aspirin can not reduce the incidence of heart disease ）
H1：π1－π2＜0（ Taking aspirin can reduce the incidence of heart disease ）
This is a one-sided test , Test statistics z by ：

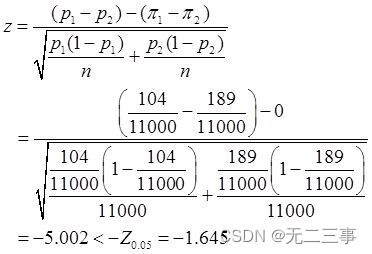
So reject the original assumption , That is, taking aspirin can reduce the incidence of heart disease .

14 A factory manufactures bolts , The specified bolt diameter is 7.0cm, The variance of 0.03cm2. Today, from a batch of bolts 80 Measure its caliber , The average is 6.97cm, The variance of 0.0375cm2. Assume that the bolt diameter follows a normal distribution , Ask whether these bolts meet the specified requirements （α＝0.05）？

Explain ： Whether these bolts meet the specified requirements , We need to test the mean and variance respectively .
（1） Make assumptions ：
H0：μ＝7.0;H1：μ≠7.0
This is a bilateral test , And the variance is known , Test statistics z by ：

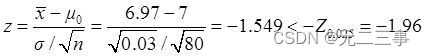
So we can't reject the original hypothesis .

 （2） Make assumptions ：
H0：σ2＝0.03;H1：σ2≠0.03
This is a bilateral test , Test statistics χ2 by ：

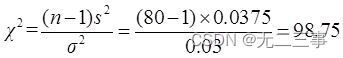
because χ0.9752（79）＝56.31＜98.75＜χ0.0252（79）＝105.5, So don't reject the original assumption .
comprehensive （1）（2） You know , These bolts meet the specified requirements .

15 Some people say that boys' academic performance is better than girls' academic performance in College . Now randomly selected from a school 25 Boys and 16 Girls , They were tested on the same topic . The test results show that , The average grade of boys is 82 branch , The variance of 562, The average grade of girls is 78 branch , The variance of 49 branch 2. Hypothetical significance level α＝0.02, What conclusions can be drawn from the above data ？

Explain ： It is necessary to judge whether the academic performance of male students is better than that of female students in University , We need to test the mean and variance respectively .
（1） Test the mean
Make assumptions ：
H0：σ12＝σ22;H1：σ12≠σ22
This is a two-sided test of two populations , Test statistics F by ：
F＝s12/s22＝56/49＝1.143
because F0.01（24,15）＝3.29,F0.99（24,15）＝0.346, be F0.99（24,15）＜F＜F0.01（24,15） So we can't reject the original hypothesis , It shows that there is no significant difference between the two populations .
（2） The other party's difference test
Make assumptions ：

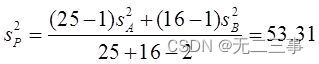
H0：μA－μB＝0;H1：μA－μB≠0
This is a two-sided test of two populations , Test statistics t by ：

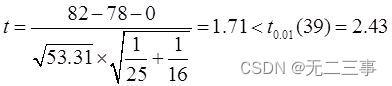
So we can't reject the original hypothesis .
comprehensive （1）（2） You know , It doesn't mean that boys' academic performance is better than girls' in College .