Statistics 8th Edition Jia Junping Chapter IX summary of knowledge points of classified data analysis and answers to exercises after class

Catalog

One 、 Knowledge framework

Two 、 After-school exercises

---

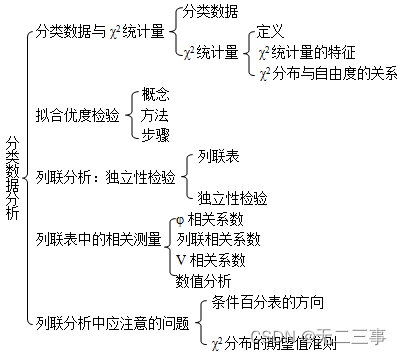
One 、 Knowledge framework

Two 、 After-school exercises

1 Market researchers want to study whether different income groups have the same buying habits for a particular commodity , They surveyed consumers in four different income groups 527 people , Buying habits are divided into ： Regular purchase , Don't buy , Sometimes I buy . The survey results are shown in the table .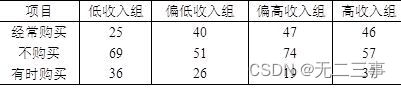

requirement ：
（1） suggest a hypothesis ;
（2） Calculation χ2 value ;
（3） With α＝0.1 To test the significance level of .

Explain ：（1） suggest a hypothesis ：
H0：π1＝π2＝π3＝π4（ That is, different income groups have the same buying habits for a particular commodity ）
H1：π1,π2,π3,π4 Not exactly equal （ That is, different income groups have different buying habits for a particular commodity ）
（2） Calculate the expected value of each group ,（ The values in brackets in the table are expected values ）.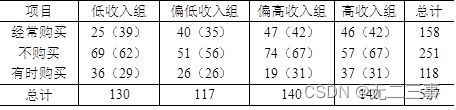

The calculation method of the expected value of each item in the table is ：
therefore χ2＝（25－39）2/39＋（69－62）2/62＋…＋（37－31）2/31＝17.67.
（3） The result of the survey is 3 That's ok 4 Contingency table of columns , Its degree of freedom ＝（3－1）×（4－1）＝6, When α＝0.1 when ,χ0.12（6）＝10.64, From the first （2） Ask the calculated χ2 The value is 17.67＞10.64＝χ0.12（6）, So we reject the original hypothesis , That is, different income groups have different buying habits for a particular commodity .

2 Randomly selected from the population n＝200 The sample of , Classified by different attributes after investigation , The results are as follows ：
n1＝28,n2＝56,n3＝48,n4＝36,n5＝32
Based on empirical data , The proportion of each category in the total is ：
π1＝0.1,π2＝0.2,π3＝0.3,π4＝0.2,π5＝0.2
With α＝0.1 To test the significance level of , Explain whether the current situation has changed compared with the empirical data （ use P value ）. Explain ： suggest a hypothesis ：H0： The situation has not changed compared with empirical data ;H1： Now the situation has changed compared with empirical data .
From known conditions, we can get χ2 The value is ：

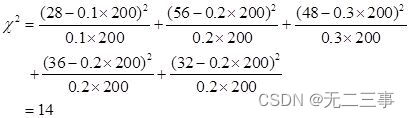
and P[χ2（5－1）＞14]＝0.007295＜0.1＝α, So we reject the original hypothesis .

3 A newspaper is concerned about whether its readers' reading habits are related to their educational level , Random investigation 254 Readers .

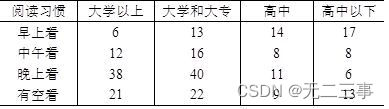
With 0.05 The significance level tests whether readers' reading habits are related to their educational level .

Explain ： Make assumptions ：
H0：π1＝π2＝π3＝π4（ That is, reading habits have nothing to do with education ）
H1：π1,π2,π3,π4 Not exactly equal （ That is, reading habits are related to educational level ）
Calculate the expectations of each group .

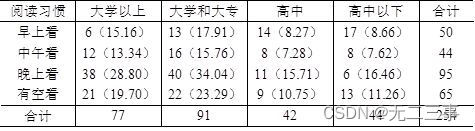
The calculation method of the expected value of each item in the table is ：
therefore χ2＝（6－15.16）2/15.16＋（12－13.34）2/13.34＋…＋（13－11.26）2/11.26＝31.86.
The survey data is 4 That's ok 4 Contingency table of columns , Its degree of freedom is ＝（4－1）×（4－1）＝9,P[χ2（9）＞31.86]＝0.0002＜0.05＝α, So we reject the original hypothesis , I think reading habits are related to educational level .

4 After the teaching reform, students have more freedom to choose courses , But college leaders also face new problems in arranging courses . for example ,MBA The course selection of students in graduate classes often changes greatly between academic years , Last year, many students took accounting courses , This year, many students choose marketing courses . Because it is impossible to determine in advance how many students choose each course , Therefore, it is impossible to prepare teaching resources effectively . It has been suggested that the courses chosen by students are related to their undergraduate majors . So , College leaders will associate students' majors with MBA Statistics have been made on the elective courses of the three courses .

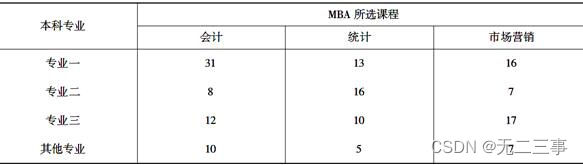
requirement ：
（1） With 0.05 The significance level tests whether the majors of undergraduate students affect their reading MBA Selected courses during ;
（2） Calculation P value .

  Explain ：（1） Make assumptions ：
H0：π1＝π2＝π3＝π4（ That is, undergraduate majors and MBA It has nothing to do with choosing courses ）
H1：π1,π2,π3,π4 Not exactly equal （ That is, undergraduate majors and MBA Relevant to course selection ）
Calculate the expectations of each group .

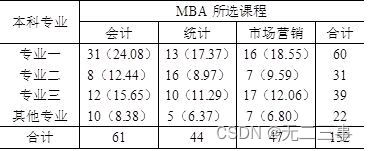
The calculation method of the expected value of each item in the table is ：
therefore χ2＝（31－24.08）2/24.08＋（8－12.44）2/12.44＋…＋（7－6.80）2/6.80＝14.71.
The statistical result of this elective course is 4 That's ok 3 Contingency table of columns , Its degree of freedom is ＝（4－1）×（3－1）＝6, When α＝0.05 when ,χ0.052（6）＝12.592,χ2＝14.71＞12.592＝χ0.052（6）, So reject the original assumption .
（2） From the first （1） The calculation result of the question can be obtained ：P[χ2（6）＞14.71]＝0.023＜0.05＝α, So we reject the original hypothesis , Think undergraduate major and MBA Relevant to course selection .