当前位置:网站首页>Statistics 8th Edition Jia Junping Chapter 5 probability and probability distribution
Statistics 8th Edition Jia Junping Chapter 5 probability and probability distribution
2022-07-06 14:29:00 【No two or three things】
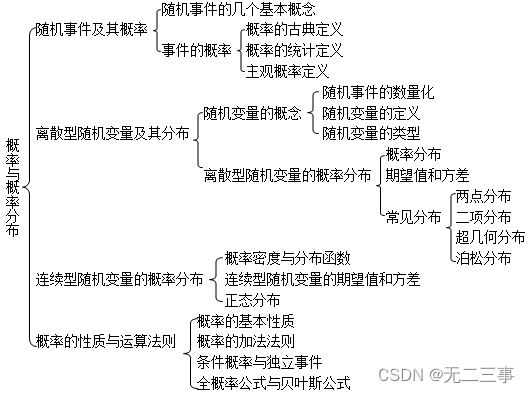
One 、 Examination site induction
Two 、 Exercises 1 Write the sample space for the following randomized trials :
(1) Record the average score of a statistical test in a class ;
(2) Someone is riding a bike on the road , Observe the number of times the cyclist meets the green light before stopping at the first red light ;
(3) Produce products until 10 Until it is genuine , Record the total number of products produced .
Explain :(1) The average score is in the range 0~100 A continuous variable between , So the sample space of the average score Ω=[0,100].
(2) The number of green lights encountered is from 0 Any natural number at the beginning , So sample space Ω=N.
(3) There may be no defective products or any number of defective products in the products previously produced , So sample space Ω={10,11,12,13,…}.
2 Someone's flower 2 Yuan to buy lottery , He hit 100 The probability of a yuan prize is 0.1%, win a lottery 10 The probability of a yuan prize is 1%, win a lottery 1 The probability of a yuan prize is 20%, Suppose all kinds of prizes cannot be won at the same time , Try to ask for :
(1) The probability distribution of this person's income .
(2) The expected value of this person's income .
Explain :(1) set up X For the benefit of this person , win a lottery 100 The income of yuan prize is 100-2=98( element ), win a lottery 10 The income of yuan prize is 10-2=8( element ), win a lottery 1 When you win a prize, you will lose 1 element , win a lottery 0 When you win a prize, you will lose 2 element , Then the probability distribution of this person's income is :
P(X=98)=0.001
P(X=8)=0.01
P(X=-1)=0.2
P(X=-2)=1-P(X=98)-P(X=8)-P(X=-1)=0.789
(2)E(X)=98×0.001+8×0.01+(-1)×0.2+(-2)×0.789=1.6
3 Set the random variable X The probability density of is :
f(x)=3x2/θ3,0<x<θ
(1) It is known that P(X>1)=7/8, seek θ Value .
(2) seek X Expectation and variance of .
Explain :(1) from P(X>1)=7/8 Available :P(X≤1)=1-P(X>1)=1/8, namely
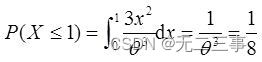
Solution :θ=2. therefore , A random variable X The probability density of is :f(x)=3x2/8,0<x<2.
(2) from X The expected value of the probability density is :
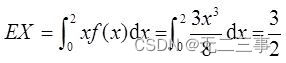
also
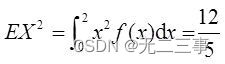
therefore
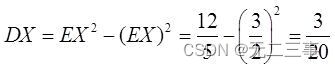
4 There is 5 questions , At the same time, each question lists 4 An alternative answer , One of the answers is correct . A student can answer correctly by guessing at least 4 What's the probability of the problem ?
Explain : set up X Is the number of correct answers . From the meaning of the question , The probability of answering a question correctly is 1/4. that X~B(5,1/4), So the answer is right, at least 4 The probability of the problem is :
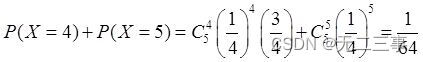
5 Set the random variable X The compliance parameter is λ The Poisson distribution of , And known P{X=1}=P{X=2}, seek P{X=4}. Explain : The formula of Poisson distribution has :
P(X=1)=λe-λ
P(X=2)=λ2e-λ/(2!)
from P{X=1}=P{X=2}, Solution :λ=2.
therefore P(X=4)=24×e-2÷4!=2/(3e2).
6 set up X~N(3,4), Try to ask for :(1)P{|X|>2};(2)P{X>3}.
Explain :(1)
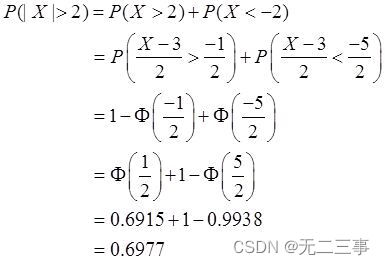
(2) because N(3,4) About the mean 3 symmetry , therefore P{X>3}=1/2.
7 The life of electronic tubes produced by a factory X( In hours ) Obey expectations μ=160 Is a normal distribution , If required P{120<X<200}≥0.08, Allowable standard deviation σ What is the maximum ?
Explain : from
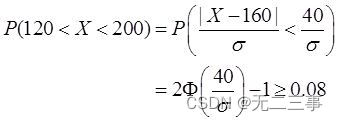
Available :Φ(40/σ)≥0.54, Look up the table :40/σ≥0.1004, so σ≤398.41. That is, the allowable standard deviation σ The maximum is 398.41.8 The number of errors in the proofreading of a book after typesetting X It follows a normal distribution N(200,400), seek :
(1) The number of errors does not exceed 230 Probability .
(2) The number of errors is 190~210 Probability between .
Explain :(1) The number of errors does not exceed 230 The probability of is :
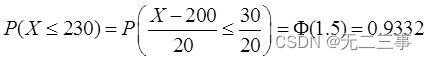
(2) The number of errors is 190~210 The probability between is :
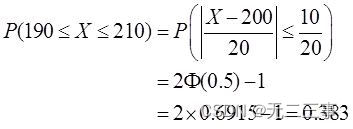
边栏推荐
- Strengthen basic learning records
- 《英特尔 oneAPI—打开异构新纪元》
- sqqyw(淡然点图标系统)漏洞复现和74cms漏洞复现
- Experiment 4 array
- Detailed explanation of network foundation routing
- 《统计学》第八版贾俊平第七章知识点总结及课后习题答案
- Wei Shen of Peking University revealed the current situation: his class is not very good, and there are only 5 or 6 middle-term students left after leaving class
- Overview of LNMP architecture and construction of related services
- . Net6: develop modern 3D industrial software based on WPF (2)
- XSS (cross site scripting attack) for security interview
猜你喜欢
JDBC transactions, batch processing, and connection pooling (super detailed)

JDBC事务、批处理以及连接池(超详细)
Internet Management (Information Collection)
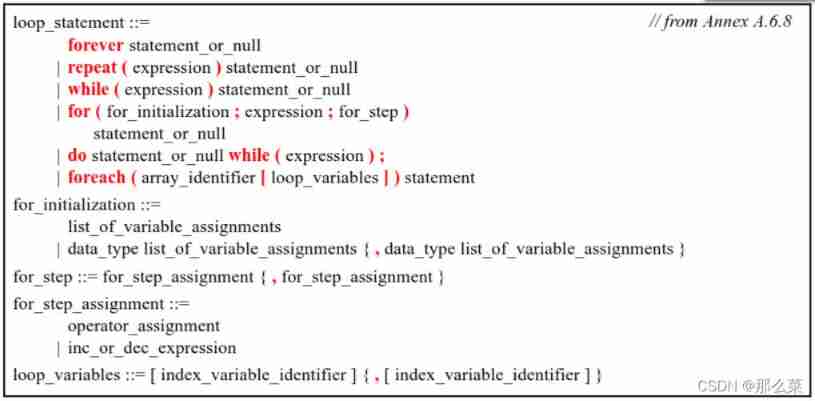
SystemVerilog discusses loop loop structure and built-in loop variable I

Hackmyvm target series (5) -warez
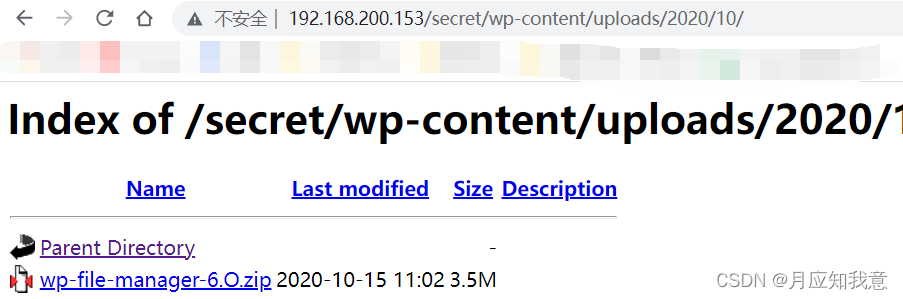
Hackmyvm target series (4) -vulny
《统计学》第八版贾俊平第三章课后习题及答案总结
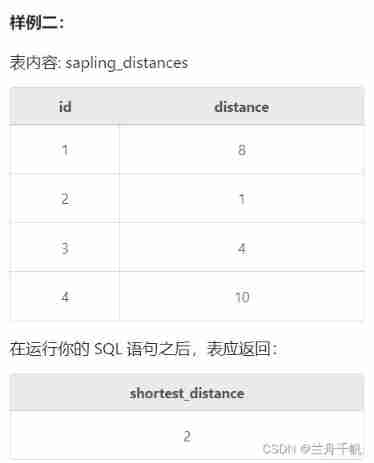
Lintcode logo queries the two nearest saplings
Intranet information collection of Intranet penetration (I)

Constants, variables, and operators of SystemVerilog usage
随机推荐
Hackmyvm target series (1) -webmaster
强化學習基礎記錄
HackMyvm靶机系列(3)-visions
How to test whether an object is a proxy- How to test if an object is a Proxy?
Wei Shen of Peking University revealed the current situation: his class is not very good, and there are only 5 or 6 middle-term students left after leaving class
DVWA (5th week)
Hackmyvm target series (3) -visions
Detailed explanation of three ways of HTTP caching
Record an API interface SQL injection practice
Strengthen basic learning records
Sword finger offer 23 - print binary tree from top to bottom
Experiment 7 use of common classes
This article explains in detail how mockmvc is used in practical work
JVM memory model concept
图书管理系统
HackMyvm靶机系列(2)-warrior
7-5 staircase upgrade (PTA program design)
Tencent map circle
Intranet information collection of Intranet penetration (5)
Overview of LNMP architecture and construction of related services